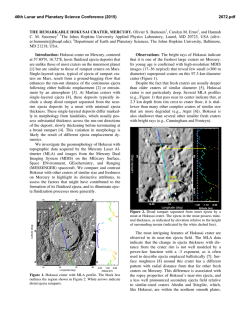
46th Lunar and Planetary Science Conference (2015) 2894.pdf EXPERIMENTAL MEASUREMENTS OF MOMENTUM TRANSFER IN HYPVELOCITY COLLISIONS. K. R. Housen and K. A. Holsapple, 1The Boeing Co., P.O. Box 3707, Seattle WA 98124, [email protected], 2Univ. Washington, 352400, Seattle WA, 98195, [email protected] Introduction: Hypervelocity collisions affect the rotational states of asteroids and have been suggested as a method for altering the orbits of potentially hazardous bodies. Both of these processes depend significantly not only on the projectile momentum, but also on the impulse contained in the excavated surface material that permanently escapes the asteroid. Given that the volume of a hypervelocity impact crater can be orders of magnitude larger than the impactor volume, and that much of the ejecta escape the weak gravity field of a small asteroid, the impulse contribution from the ejecta can be significantly larger than the direct contribution from the impactor. The efficiency of this process is characterized by a parameter, β, defined as the total momentum change of the asteroid divided by the momentum of the impacting projectile. In the limiting case of a perfectly inelastic collision (no ejecta), β=1. When a considerable mass of ejecta escapes, β can be significantly greater than 1. We use experiments, scaling analysis and shock wave codes (hydrocodes) to study the momentum transfer for a deflection mission. The purpose of this abstract is to summarize our recent experiments. Experiments: The impact experiments are performed in the Boeing Shock Physics Lab, as well as at the NASA Ames Vertical Gun Range. As described in previous reports (1), the target container is suspended in an evacuated impact chamber by four steel springs whose attachment points are equally spaced around the perimeter of the bowl-shaped container. The impacts occur vertically, normal to the target surface. The projectiles are either polyethylene cylinders or aluminum or nylon spheres. The impact velocity ranges from 0.5 to 5.7 km/s. A Keyence laser displacement gage aimed at the bottom of the target container measures the vertical oscillations of the target caused by the impact. The laser records determine the frequency ω and amplitude H of the oscillations. The impulse delivered to the target is HωM/2, where M is the mass of the target. That impulse, divided by the initial projectile momentum, gives the value of β for the experiment. Asteroids and comets exhibit a rich diversity of shapes, bulk densities, reflection spectra and surface features. This undoubtedly reflects a corresponding diversity in the mechancial properties of the surface materials, which affect the amount and speed of material ejected during a collision. Our approach is to measure β for a wide variety of target material types and configurations to reveal the range of β that could be expected during hypervelocity collision events. The target materials include dry sand, various types of nonporous rock (monolithic blocks of Columbia basalt and High Sierra granite, and “river rock”, obtained from a landscape supplier), coherent pumice with density=0.87 gm/cm3 and ~70% porosity, granular pumice with a bulk density of 84%, various sizes of basalt gravel, and simulants of regolith-covered asteroids consisting of a layer of sand over competent rock. Results: The point-source theory of hypervelocity impact indicates that β should generally increase with impact speed (2). In particular, β-1, i.e. the contribution from the ejecta, should increase as a power of speed. The figure below shows our current set of experimental results as a log-log plot of β-1 vs impact speed U, in which case the power-law dependence becomes a straight line. We find that competent, nonporous rock exhibits the highest value of β, due to the high ejecta velocities observed for these materials. Extrapolating the observed power-law dependence, β could be as large as 10 at speeds of 15 to 20 km/s. At the other extreme, highly porous materials, such as pumice, tend to show much lower values of β, often close to the inelastic limit of β =1. This is due to the rapid decay of the shock in porous materials, and correspondingly low ejection velocities (3). Granular materials, such as sand or gravel, lie between these two cases. Dry sand targets (green data points) have small β at the low impact speeds, but could reach 4 to 5 at speeds of 15 to 20 km/s. Impacts into gravel targets, meant as rough analogs of blocky rubble-pile asteroids, fall between those of sand and competent rock. The large gray squares in the figure are for coarse gravel targets where the gravel particles (~45 mm dia) where about 7x larger than the aluminum projectile. The smaller gray squares correspond to two finer sizes of gravel, the finest having particle sizes comparable to the projectile. The results for fine gravel approach those for sand, as expected. Increasing the constituent particle size generally produces larger values of β, and would presumably approach the competent rock line for sufficiently large particles. 46th Lunar and Planetary Science Conference (2015) We have performed four experiments with targets consisting of a layer of dry sand on top of a block of competent basalt (small blue squares). The line connecting the two points in the figure corresponds to a layer that is four projectile diameters deep. When the regolith depth is doubled, β increases noticeably (the highest small blue square). A further increase in the layer depth causes a reduction in β. This is expected because for sufficiently deep layers, the projectile and 2894.pdf the cratering flow is not affected by the deeply buried rock layer. Further results and implications of these experiments will be presented. References: [1] Housen K.R. and Holsapple K.A. (2011) 42nd Lunar Planet. Sci. Conf., 2363. [2] (1) Holsapple, K. and Housen, K. (2012) Icarus, 221, 875887. [3] Housen and Holsapple (2011), Icarus 211, 856-875. Figure 1. Experimental measurements of the momentum enhancement factor, β.