Download Report

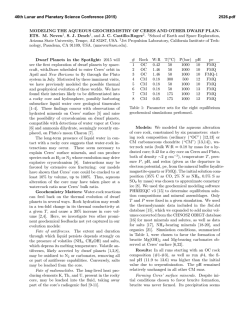
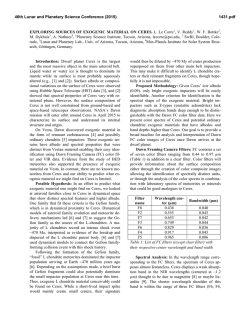
46th Lunar and Planetary Science Conference (2015)
1418.pdf
A PRELIMINARY CHRONOLOGY FOR CERES. N. Schmedemann1, G. Michael1, B. A. Ivanov2, T. Kneissl1,
A. Neesemann1, H. Hiesinger3, R. Jaumann1,4, C. A. Raymond5, C. T. Russell6, 1Institute of Geological Sciences,
Freie Universität Berlin, Berlin, Germany, 2Institute of Dynamics of Geospheres, Moscow, Russia, 3Institut für Planetologie, Westfälische Wilhelms-Universität, Münster, Germany, 4German Aerospace Center, Institute of Planetary
Research, Berlin, Germany 5JPL, Caltech, Pasadena, CA, USA, 6University of California, Los Angeles, CA, USA.
([email protected])
Introduction: The highly successful Dawn mission
[1] finished data collection at Vesta in 2012 and is now
on its way to the dwarf planet Ceres, where it will arrive
in spring 2015. The determination of crater retention
ages on Vesta was carried out using two different chronology systems [2] that led to significantly different results. There is not just one root source for the disagreement but three of them concerning the geological interpretation of measurement areas, the crater production
function and the chronology function [3]. Each disagreement contributes individually variable differences
depending on the area of measurement and its geological interpretation. The final version of the lunar-like
chronology system (geol. interpretation + production
function + chronology function) for Vesta [3] for instance is able to provide absolute ages for the formation
of the Rheasilvia basin on Vesta in reasonable agreement with observed relative stratigraphy and independent radiometric ages of Vesta derived brecciated HED
meteorites [4], which probably recorded the Rheasilvia
impact event. Here we present a preliminary version of
a lunar-like chronology for Ceres. Different from the
case of Vesta there are no known meteorites that unambiguously originate from Ceres. Thus, cross-checking
with ground truth data is not yet possible. The Ceres
chronology we present here will be updated as Dawn
provides more accurate gravity data and high resolution
imaging data, from which the transition diameter from
simple to complex craters will be measured directly.
This value is crucial for our crater scaling calculation.
Methodology: The derivation of a crater production
function and chronology function contains three steps.
I. Estimation of average impact velocities and impact probabilities for Ceres: This is done by a statistical
analysis of the orbit geometries of Ceres crossing asteroids [5,6]. For the intrinsic impact probability of Ceres,
we derive from crossing bodies ≥ 5 km diameter a value
of 2.84x10-18 km-2a-1. The average impact velocity is
calculated at about 4.6 km/s. Fig.1 shows the distribution of impact probabilities vs. impact velocities for
Vesta and Ceres.
II. Derivation of the lunar-like crater production
function: For Ceres we assume the same projectile distribution that impacted the Moon. The reasoning for this
approach is given in [3]. It might be possible that there
is a slight difference in the projectile distribution of the
Moon and Ceres for projectile diameters > 10 km. This
should not affect the calibration of the chronology in
step 3 but could lead to slightly different results in crater
retention ages using craters > ~ 80 km.
Fig. 1: Impact probability vs. impact velocity diagram for
Ceres and Vesta.
In order to scale the lunar crater production function to
the impact conditions on Ceres we use the Ivanov scaling laws [7, Eq 1].
.
.
.
(1)
.
Dt is the diameter of the transient crater and DP is the
diameter of the projectiles. For simple craters the transient crater diameter is nearly the same as the final crater
diameter. [7] gives a second equation for computing the
diameter of complex craters from the transient crater diameter. Table 1 gives an overview of the scaling parameters we used for the Moon and Ceres.
Table 1: Scaling parameters for the Moon and Ceres.
Parameter Moon Ceres ρ; target density [g/cm³] δ; projectile density [g/cm³] v; impact velocity [km/s] α; impact angle [degree] g; surface gravity [m/s²] sg; strength‐gravity trans. [km] sc; simple‐complex trans. [km] 1.8 [3] 1 2 [3] 2 [3] 17.5 [3] (4.57²+0.51²)(1/2) 45 [3] 45 [3] 1.62 [7] 0.276 [8] (1.444) 0.3 [7] 0.336 15 [7] 12.12 46th Lunar and Planetary Science Conference (2015)
It is assumed that up to ~100 km below Ceres’ surface
a water-ice layer exists [8]. Thus, we have to consider
the different crater-scaling going from the basaltic lunar/Vestan surfaces to water ice at Ceres. Therefore, we
use 1 g/cm³ as the target density and scale the simple to
complex transition from the icy Saturnian satellite Iapetus (15 km; [9]) via a 1/g approach [10] to Ceres. Different surface temparatures (ΔT ~70K) of both bodies
may result in slightly different scaling behavior. From
Eq (2) we use Vesta to get an estimate for the strength
to gravity transition at Ceres. Eq (3) provides a modified
surface gravity for Ceres that accounts for the different
scaling behavior of water ice compared to the basaltic
target material found on Vesta (Vesta values are taken
from [3]).
/
/
(2);
(3)
Table 1 gives the modified surface gravity for Ceres in
brackets. This value is used for scaling the crater production function. Fig. 2 gives a comparison between the
lunar, Vestan and Cerean crater production function.
1418.pdf
production function with the existing cratering record.
At the time of the presentation initial measurements of
the Cerean crater size-frequency distribution may allow
for the first evaluation of the quality of the derived functions. The yet to be observed simple to complex transition size is crucial to our approach. Another issue could
be that the derived crater production function may
slightly differ from the observed crater size distribution
due to relatively rapid crater modification in ice. Thus,
the derived functions will very likely be improved when
Dawn arrives at Ceres.
Table 2: Coefficients for the polynomial function of 11th degree for the Moon, Vesta and Ceres.
Moon
Vesta
Ceres
[11]
Rev4 [3]
−2.5489
−2.8783
-3.1085
a0
−2.9794
−2.8687
-3.3594
a1
0.42605
0.53853
0.76227
a2
0.32288
0.30803
0.76669
a3
−0.030823
−0.048714
-0.13398
a4
−0.022295
−0.018894
-0.31221
a5
0.019473
0.00371
-0.0055569
a6
−0.022278
−0.025344
0.047208
a7
−0.0085611
−0.0033966
0.0016841
a8
0.0053854
0.005711
5.0702e-05
a9
0.00087331
0.0004281
-0.001079
a10
−0.0003887
−0.00036638
8.0452e-05
a11
Table 3: Coefficients for lunar-like chronology for the Moon,
Vesta and Ceres.
C1
C2
C3
Moon
[11]
Vesta
rev4 [3]
Ceres
5.44×10−14
6.93
8.38×10−4
1.29×10−12
6.93
1.98×10−2
1.53×10−12
6.93
2.24×10−2
References: [1] Russell C.T. et al. (2012) Science, 336, 684686; [2] Williams D. A. et al. (2014) Icarus, 244, 158-165; [3] Schmedemann N. et al. (2014), 103, 104-130; [4] Bogard D.D. (2011) Chemie der Erde, 71, 207-226; [5] Öpik E.J. (1960) Mon. Not. R. Astron.
Soc., 120, 404-411; [6] Bottke W.F. et al. (1994) Icarus, 107, 255268; [7] Ivanov B.A. (2001) Space Science Reviews, 96, 87-104; [8]
McCord T.B. et al. (2012) Ceres: Its Origin, Evolution and Structure
and Dawn’s Potential Contribution. In: Russell, C.T, Raymond, C.A.
Fig 2: Crater production functions for the Moon [11], Vesta
[3] and Ceres.
(eds.) The Dawn Mission to Minor Planets 4 Vesta and 1 Ceres.
Table 2 gives the respective coefficients for the polynomial function of 11th degree [11].
III. Determination of the Chronology Function: Using the impact probability from point I. and the approach by [3], we find for the coefficients of the lunarlike chronology (Eq 4; [11]) for Ceres the values listed
in Table 3.
263-289; [10] Pike R.J. (1980) Icarus, 43, 1-19; [11] Neukum G. and
1
1
(4)
Results: At the time of writing, Ceres was not yet
imaged with sufficient resolution to compare our crater
Springer, New York, 63-76; [9] Zahnle K. et al. (2003) Icarus, 163,
Ivanov B. A. (1994) Crater size distributions and impact probabilities
on Earth from Lunar, terrestrial planet, and asteroid cratering data. In:
Gehrels T. (ed) Hazards due to comets and asteroids. University of
Arizona Press, Tucson, 359–416.
Acknowledgments: This work has been supported by the
German Space Agency (DLR) on behalf of the Federal Ministry of
Economic Affairs and Energy, grants 50OW1101 (NS, TK, AN) and
50QM1301 (GM). BAI is supported by Program 22 RAS.