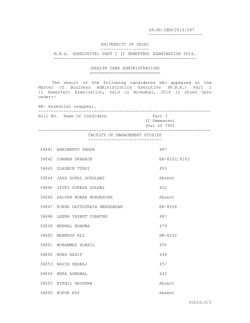
a priori judgments
Philosophy 190: Seminar on Kant Spring, 2015 Prof. Peter Hadreas Course website: http://oucampus.sjsu.edu/people/peter.hadreas/courses/Ka nt/index.html NOTE: The Guyer and Wood translation of the Critique of Pure Reason – the text we’re using -- can be accessed online at http://strangebeautiful.com/lmu/readings/ka nt-first-critique-cambridge.pdf Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. Very Short Historical Review of the a prioria posteriori distinction In Metaphysics, Book V, Chapter 11, Aristotle itemizes five senses of prior: two in respect of knowledge, in respect of nature and substance, and one in respect of attributes per se. Scholasticism dominated medieval universities in Europe from about 1100 to 1700. Picture on the right is a 14th-century fresco of a university lecture. In the Scholastic tradition,, i. e. the medieval appropriation of Aristotle, the terms literally mean "from what is prior" and "from what is posterior." In the Scholastic tradition these terms meant either: a) A is prior to B in nature only if A could not exist without B; or, b) A is prior to B in knowledge only if we cannot know A without knowing B. The a priori - a posteriori distinction as it was taught in Kant’s day. In the Leibnizian-Wolff system which was the philosophy "of the Schools" in Kant's day, a priori and a posteriori were define according to causal relations: "When the mind reasons from causes to effect, the demonstration is called a priori; when from effects to causes, the demonstration is called a posteriori.” It was this usage which Hume criticizes in the Treatise of Human Nature, Book I. (1739). It follows from Hume's analysis of causation that if there is no necessary connection between cause and effect, the distinction between a priori and a posteriori falls apart. Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. “In the sequel therefore we will understand by a priori cognitions not those that occur independently of this or that experience, but rather those that occur absolutely independently of all experience. Opposed to them are empirical cognitions, or those that are possible only a posteriori, i.e., through experience. Among a priori cognitions, however, those are called pure with which nothing empirical is intermixed. Thus, e.g., the proposition "Every alteration has its cause" is an a priori proposition, only not pure, since alteration is a concept that can be drawn only from experience.” (p. 117) Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. “First, then, if a proposition is thought along with its necessity, it is an a priori judgment; if it is, moreover, also not derived from any proposition except one that in turn is valid as a necessary proposition, then it is absolutely a priori. Second: Experience never gives its judgments true or strict but only assumed and comparative universality (through induction), so properly it must be said: as far as we have yet perceived, there is no exception to this or that rule. Thus if a judgment is thought in strict universality, i.e., in such a way that no exception at all is allowed to be possible, then it is not derived from experience, but is rather valid absolutely a priori.” Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. So necessary conditions of a all priori judgments are that they are necessary and universal. Using Kant’s examples: “Every body has a cause” is a priori. “All bodies are heavy” is not a priori. In these examples Kant’s transcendental method is already at work. “Gradually remove from your experiential concept of a body everything this is empirical in it – the color, the hardness, or softness, even the impenetrability – there still remains the space that was occupied by the body (which has now entirely disappeared), and you cannot leave that out.” (p. 138) Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. “All prime numbers greater than two are odd.” An priori judgment. It necessary and universal. “All U. S. Presidents are male.” Not a priori. True, but neither necessary nor strictly universal. There could be a U. S. President who is not male. There may be in 2017. It is an a posteriori judgment. Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. A very notorious example of a mathematical claim that was thought to be a priori but was finally determined not to be: Euclid’s Fifth Postulate: “If a line segment intersects two straight lines forming two interior angles on the same side that sum to less than two right angles, then the two lines, if extended indefinitely, meet on that side on which the angles sum to less than two right angles.” Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136152. On the difference between pure and empirical cognition. [Kant Exposes Epistemological Fallacy #1 ”There is no doubt whatever that all our cognition begins with experience; for how else should the cognitive faculty be awakened into exercise if not through objects that stimulate our senses and in part themselves produce representations, in part bring the activity of our understanding into motion to compare these, to connect or separate them, and thus to work up the raw material of sensible impressions into a cognition of objects that is called experience?” (p. 136) [continues] Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. Kant Exposes Epistemological Fallacy #1 “As far as time is concerned, then, no cognition in us precedes experience, and with experience every cognition begins. But although all our cognition commences with experience, yet it does not on that account all arise from experience.1 For it could well be that even our experiential cognition is a composite of that which we receive through impressions and that which our own cognitive faculty (merely prompted by sensible impressions) provides out of itself, which addition we cannot distinguish from that fundamental material until long practice has made us attentive to it and skilled in separating it out.” (p. 136) Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. Fundamental epistemological fallacy #1. “But although all our cognition commences with experience, yet it does not on that account all arise from experience.” (p. 136) This fallacy is directed particularly at empiricists. Introduction to the Second Edition of the Critique of Pure Reason (1787), pp. 136-152. Kant Exposes Epistemological Fallacy #2 The Extension of a priori reasoning beyond sensory experience. The fallacy is particularly directed at the rationalists, especially Leibniz, Wolff, and when applied to non-ethical issues, Plato. “Captivated by such a proof of the power of reason, the drive for expansion sees no bounds. The light dove, in free flight cutting through the air the resistance of which it feels, could get the idea that it could do even better in airless space. Likewise, Plato abandoned the world of the senses because it set such narrow limits for the understanding, and dared to go beyond it on the wings of the ideas, in the empty space of pure understanding.” (p. 140.) Kant’s Use of a priori is multi-faceted. Kant applies the notion of a priori 1) to knowledge, 2) judgments, 3) concepts, 4) ‘principles,' and 5) a faculty of a priori knowledge.1 1. CPR B4: Necessity and strict universality are thus sure criteria of a priori knowledge, and are inseparable from one another. CPR B4: "it is easy to show that there actually are in human knowledge judgments which are necessary and in the strictest sense universal, and which are therefore pure a priori judgments'. CPR B5: it is possible to show that pure a priori principles are indispensable for the possibility of experience, and so to prove their existence a priori. For when could experience derive its certainty, if all the rules, according to which it proceeds, were always themselves empirical, and therefore contingent. CPR B5): Such a priori origin is manifest in certain concepts, no less than judgment." [Kant gives the example that a corporeal body implies a space which it occupies.] CPR B6: "Owing, therefore to the necessity with which this concept of substance forces itself upon us, we have no option save to admit that it has its seat in our faculty of a priori knowledge." (See also B4: 'a faculty of a priori knowledge.' Kant’s Introduction of the ‘analytic’/ ‘synthetic Distinction The distinction between 'analytic' and 'synthetic' judgments was developed by Kant himself and developed especially out of his criticism of the Leibniz-Wolffian school. Leibniz treated all judgments as analytic. “In all judgments in which the relation of a subject to the predicate is . . . this relation is possible in two different ways. Either the predicate B belongs to the subject A as something that is (covertly) contained in this concept A; or B lies entirely outside the concept A, though to be sure it stands in connection with it. In the first case I call the judgment analytic, in the second synthetic.” (p. 141) [continued] Kant’s Introduction of the ‘analytic’/ ‘synthetic Distinction “Analytic judgments (affirmative ones) are thus those in which the connection of the predicate is thought through identity, but those in which this connection is thought without identity are to be called synthetic judgments. One could also call the former judgments of clarification, and the latter judgments of amplification since through the predicate the former do not add anything to the concept of the subject, but only break it up by means of analysis into its component concepts, which were already thought in it (though confusedly); while the latter, on the contrary, add to the concept of the subject a predicate that was not thought in it at all, and could not have been extracted from it through any analysis.” (p. 141) [continued] Kant’s Introduction of the ‘analytic’/ ‘synthetic Distinction [continued from previous slide] “E.g., if I say: "All bodies are extended," then this is an analytic judgment. For I do not need to go beyond the concept that I combine with the body in order to find that extension is connected with it, but rather I need only to analyze that concept, i.e., become conscious of the manifold that I always think in it, in order to encounter this predicate therein; it is therefore an analytic judgment. On the contrary, if I say: "All bodies are heavy," then the predicate is something entirely different from that which I think in the mere concept of a body in general. The addition of such a predicate thus yields a synthetic judgment.” (p. 141) [continues} Kant’s Introduction of the ‘analytic’/ ‘synthetic Distinction [continued from previous slide] “Judgments of experience, as such, are all synthetic. For it would be absurd to ground an analytic judgment on experience, since I do not need to go beyond my concept at all in order to formulate the judgment, and therefore need no testimony from experience for that.” (p. 142) The Key Question of Division One of the CPR: How are synthetic a priori judgments possible? Kant’s allows a few intuitively obvious mathematical truths to be analytic. His examples: “To be sure, a few principles that the geometers presuppose are actually analytic and rest on the principle of contradiction; but they also only serve, as identical propositions, for the chain of method and not as principles, e.g., a = a, the whole is equal to itself, or (a + b) > a, i.e., the whole is greater than its part. And yet even these, although they are valid in accordance with mere concepts, are admitted in mathematics only because they can be exhibited in intuition.” (p. 145) But all but the most intuitively obvious true proposition of mathematics and science are synthetic a priori judgments. Even 7 + 5 = 12 is synthetic a priori. “The arithmetical proposition is therefore always synthetic; one becomes all the more distinctly aware of that if one takes somewhat larger numbers, for it is then clear that, twist and turn our concepts as we will, without getting help from intuition we could never find the sum by means of the mere analysis of our concepts.” (p. 144) All but the most intuitively obvious true propositions of mathematics and science are synthetic a priori judgments. “A straight line is the shortest distance between two points” is synthetic a priori. “That the straight line between two points is the shortest is a synthetic proposition. For my concept of the straight contains nothing of quantity, but only a quality. The concept of the shortest is therefore entirely additional to it, and cannot be extracted out of the concept of the straight line by any analysis. Help must here be gotten from intuition, by means of which alone the synthesis is possible.” (p. 145) A definition of ‘straight line’ relies on concepts outside of those contained in ‘straight’ and ‘line’ themselves. Some definitions of straight line. 1. Plato, Parmenides 137 E. And the straight, again, is that of which the middle is in the nearest line between the two extremes." 2. Euclid, Elements, Book One, Definition #4: "A straight line is a line which lies evenly with the points on itself.” 3. Archimedes (c. 225 B. C.), On the Sphere and the Cylinder, Book I, Assumptions, "Of all lines which have the same extremities the straight line is the least." 4. Carl Friedrich Gauss, (1777 –1855). "The line in which lie all points that, during the revolution of a body (or part of space) about two fixed points, maintain their position unchanged is called a straight line." Kant’s Examples of Synthetic A Priori Judgments from Physical Sciences “Natural science (Physica) contains within itself synthetic a priori judgments as principles. I will adduce only a couple of propositions as examples, such as the proposition that in all alterations of the corporeal world the quantity of matter remains unaltered, [the law of the conservation of mass]1 or that in all communication of motion effect and counter-effect must always be equal.2 In both of these not only the necessity, thus their a priori origin, but also that they are synthetic propositions is clear.” (p. 145) 1. Mass conservation was discovered in chemical reactions by Antoine Lavoisier in the late 18th century. This law contributed to the progress from alchemy to the modern natural science of chemistry. 2. Newton’s Third Law of Motion. Kant’s Examples of Synthetic A Priori Judgments from Metaphysics “In metaphysics, even if one regards it as a science that has thus far merely been sought but is nevertheless indispensable because of the nature of human reason, synthetic a priori cognitions are supposed to be contained, and it is not concerned merely with analyzing concepts that we make of things a priori and thereby clarifying them analytically, but we want to amplify our cognition a priori; to this end we must make use of such principles that add something to the given concepts that was not contained in them, and through synthetic a priori judgments go so far beyond that experience itself cannot follow us that far, e.g., in the proposition "The world must have a first beginning," and others besides, and thus metaphysics, at least as far as its end is concerned, consists of purely synthetic a priori propositions.” (pp. 145-6) Will the CPR be an ‘Organon’ or a ‘Canon’? VII. Section VII of 'The Introduction’, “The Idea and Division of a Special Science, under the Title 'Critique of Pure Reason'" (p. 149, B 24-5) "An organon of pure reason would be the sum total of those principles in accordance with which all modes of pure a priori knowledge can be acquired and actually brought about.” . . . . . (p. 150, B26.) “Such a critique is accordingly a preparation, if possible, for an organon, and, if this cannot be accomplished, then at least for a canon, in accordance with with which the complete system of philosophy of pure reason, whether it is to consist in the amplification or the mere limitation of its of its cognition, can at least some day be established both analytically and synthetically.” Main Historical Developments Since Kant Which Have Set Out to Establish New Foundation the Synthetic A Priori Issue. Gottlob Frege 1848 – 26 July 1925) Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. “Philosophical motives have prompted me to enquiries of this kind. The answers to the questions raised about the nature of arithmetical truths -- are they a priori or a posteriori? synthetic or analytic? -- must lie in this same direction. For even though the concepts concerned may themselves belong to philosophy, yet, as I believe, no decision on these questions can be reached without assistance from mathematics -- though this depends on the sense in which we understand them.” Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. ”It not uncommonly happens that we first discover the content of a proposition, and only later give the rigorous proof of it, on other and more difficult lines; and often this same proof also reveals more precisely the conditions restricting the validity of the original proposition. In general therefore, the question of how we arrive at the content of a judgment should be kept distinct from the other question. Whence do we derive the justification for its assertion?” Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. “Now distinctions between a priori and a posteriori, synthetic and analytic, concern, as I see it,1 not the content of the judgment, but the justification for making the judgment.” 1. By this I do not, of course, mean to assign a new sense to these terms, but only to state accurately what earlier writers, Kant in particular, have meant by them. Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. This means that the question is removed from the sphere of psychology, and assigned, if the truth concerned is a mathematical one, to the sphere of mathematics. The problem becomes, in fact, that of finding the proof of the proposition, and of following it up right back to the primitive truths. If, in carrying out this process, we come only on general logical laws and on definitions, then the truth is an analytic one, bearing in mind that we must take account also of all propositions upon which the admissibility of any of the definitions depends. Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. “If, however, it is impossible to give the proof without making use of truths which are not of a general logical nature, but belong to the sphere of some special science, then the proposition is a synthetic one. For a truth to be a posteriori, it must be impossible to construct a proof of it without including an appeal to facts, i. e., to truths which cannot be proved and are not general, since they contain assertions about particular objects. But, if on the contrary, its proof can be derived exclusively from general laws, which themselves neither need nor admit of proof, then the truth is a priori.” Frege, Gottlob, The Foundations of Arithmetic, 1884, pp. 3ff. Thus Frege opens up the notion of ‘analytic’ to include all those truths that may be inferred logically from given principles. This would undo Kant’s notion of synthetic a priori. For a judgment to be a priori it must only qualify as analytic and this means that it can be derived logically from accepted first principles. Principia Mathematica Bertrand Russell (18721970) and A.N. Whitehead (1861-1947) Frege’s concept of ‘analytic’ had a great influence on the foundations of so-called ‘analytic philosophy.’ In fact it motivated Russell and Whitehead to attempt to found all of mathematics upon logical principles. In 1903, the book had the more modest English title. Principles of Mathematics. In 1910-1913 Russell and Whitehead attempted to accomplish the derivation or at least show how to do it. The three-volume magnum opus took on the weightier title Principia Mathematica. Kurt Godel’s Incompleteness Proof (1931) But Russell’s and Whitehead’s painstaking effort to found mathematics on logic, which involved many years of their lives, turned out to be in vain. The Göttigen mathematician, Hilbert, was dedicated to clarifying the foundations of geometry. He challenged mathematicians to prove rigorously that Russell and Whitehead ha succeeded. The question was finally settled in 1931 when Kurt Gödel, in a revolutionary theorem – the Incompleteness Proof– proved that in a mathematical theory of sufficient complexity – such as number theory – there must be a theorem that cannot be proven true or false. Willard Van Orman Quine (1908 –2000) Quine Attacks the Analytic/ Synthetic Distinction in a Three Main Ways in Different Developments of His Thought In “Two Dogmas of Empiricism” a highly influential chapter in his book From a Logical Point of View, reprinted by Harvard University Press in 1980, Quine suggests a weaker argument that there is a gray area between analytic and synthetic statements, so we cannot always tell whether a statement is analytic or synthetic. Consider the claim that the heights of a group of males or females will follow the frequencies described by a normal distribution, or bell curve. Is this analytic or synthetic? Quine Attacks the Analytic/ Synthetic Distinction in a Three Main Ways in Different Developments of His Thought Quine’s most influential and complex attempt to undermine the notion of analyticity turns on pressing the point that, at least in its Kantian expression, analyticity is semantic. Kant had said that a statement is analytic if the predicate is contained in the subject and Kant meant the meaning (semantic value) of the judgment. In Word and Object, (1960) Quine argues for ‘radical indeterminacy.’ The is a thought experiment which leads to the conclusion that as long as we are dealing only with the meaning (semantic value) of objects, and not their extension, we can never be certain about a translation from one language to another. Consequently the underpinnings of ‘analyticity’ are undone, because they are semantic (intentional) and not extensional. Quine Attacks the Analytic/ Synthetic Distinction in a Three Main Ways in Different Developments of His Thought Kantian Graham Bird answers Quine on this attempt to undo the groundwork of ‘analyticity’ through the thought – experiment of the radical indeterminacy of translation: “It is quite possible then to distinguish the philosophical argument from the practice that allows it. . . . We can simply allow the analytic/synthetic distinction to be used in practice independently of the philosophical argument, just as we may still use the resources of a current, perhaps inadequate, semantic theory to separate analytic from synthetic judgments or truths.”1 1. Bird,. Graham, The Revolutionary Kant, (Chicago/La Salle, IL.: Open Court, 2006), p. 61 Quine Attacks the Analytic/ Synthetic Distinction in a Three Main Ways in Different Developments of His Thought Third, sometimes Quine attacks the ‘analytic/synthetic distinction by saying that if there are enough strong theories which lead us to believe in their truth, we will give up even the most basic analytic judgments, such as the Law of Excluded Middle. We do something like this in attempting to find a model for features of quanta. It would seem that quanta have the property to be and not to be at the same place at the same time. So, the predictive value of quantum theory (presumably synthetic truths) trumps analytic truth of the Law of Excluded Middle. This is sometimes called the epistemic vulnerability of analytic truths. 1. adapted from Bird,. Graham, The Revolutionary Kant, (Chicago/La Salle, IL.: Open Court, 2006), p. 61. Quine Attacks the Analytic/ Synthetic Distinction in a Three Main Ways in Different Developments of His Thought Graham Bird answers Quine on the epistemic vulnerability of analytic truths. “For Quine even logical truths, and those elsewhere classified as analytic, are vulnerable to and can be replaced in the light of, such recalcitrant experience. Even if this is true, however, what it shows is that both analytic and synthetic truths have one such feature, epistemic vulnerability, in common. But even if they cannot be discriminated on that basis it does not follow that there is no other way of distinguishing them.”1 1. Bird,. Graham, The Revolutionary Kant, (Chicago/La Salle, IL.: Open Court, 2006), pp. 61-2. Edmund Husserl 1859-1938 Husserl’s approach is to stay with meaning. All extension can be derived from intensions for Husserl. Husserl focuses particularly on the a priori which he finds in objects and states of affairs as well as judgments. He coins the term eidos for the essence of a thing. It has elements of necessity and universality – as given to consciousness. From, Husserl, Ideas I, Section 20, (1913): “When it is actually natural science that speaks, we listen gladly and as disciples. But it is not always natural science that speaks when natural scientists are speaking; and it assuredly is not when they are talking about "philosophy of Nature" and "epistemology as a natural science.” [continues] [continued] “And, above all, it is not natural science that speaks when they try to make us believe that general truisms such as all axioms express (propositions such as "a + 1 = 1 + a", "a judgment cannot be colored", "of only two qualitatively different tones, one is lower and the other higher", "a perception is, in itself, a perception of something") are indeed expressions of experiential matters of fact; whereas we know with full insight that propositions such as those give explicative expression to data of eidetic intuition.” “But this very situation makes it clear to us that the "positivists" sometimes confuse the cardinal differences among kinds of intuition and sometimes indeed see them in contrast but, bound by their prejudices, will to accept only a single one of them as valid or even as existent. (Husserl 1913, section 20)” from “Philosophy as Rigorous Science”, (Husserl 1965, pp. 90-91) “The spell of the naturalistic point of view...has blocked the road to a great science unparalleled in its fecundity... The spell of inborn naturalism also consists in the fact that it makes it so difficult for all of us to see "essences", or "ideas" -- or rather, since in fact we do, so to speak, constantly see them, for us to let them have the peculiar value which is theirs instead of absurdly naturalizing them. Intuiting essences conceals no more difficulties or "mystical" secrets than does perception.” Slides #1, 3, 4, 5, Portrait of Immanuel Kant in mid-life: http://www.lancaster.ac.uk/users/philosophy/courses/100/Kant003.jpg Slide # 15 , bust of Aristotle: http://en.wikipedia.org/wiki/Aristotle#mediaviewer/File:Aristotle_Altemp s_Inv8575.jpg Slide #16, image of a 14th century University lecture: http://upload.wikimedia.org/wikipedia/commons/f/fc/Laurentius_de_Volt olina_001.jpg Slide #29, photograph of Gottlob Frege: https://www.google.com/search?q=frege&biw= slide #36: photograsph of Russel and Whitehead: http://www.storyofmathematics.com/20th_russell.html Slide #38: Photograph of Willard Van Orman Quine: http://www.bing.com/images/search?q=picture+of+willard+van+orman+ quine&qpvt Side #44, photograph of Edmund Husserl: http://www.husserlpage.com/
© Copyright 2025