1 limites y continuidad
límites y continuidad
007
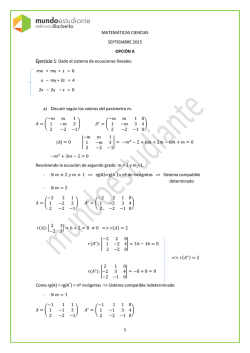
f (: x ) =
Y
x 3 − 3x
2
1
lim
x3 − 3 x
= −`
2
lim
x3 − 3 x
= +`
2
x → −`
X
1
008
0
observa la gráfica y calcula los límites de la función en el infinito.
x → +`
Busca funciones cuyos límites sean los siguientes.
a)
b)
c)
lim f ( x ) = +`
d)
lim f ( x ) = −`
e)
lim f ( x ) = +`
f)
x → +`
x → +`
x → −`
lim f ( x ) = −`
0
x → −`
lim f ( x ) no existe.
x → +`
lim f ( x ) no existe.
x → −`
Respuesta abierta. Por ejemplo:
a) f ( x ) = x 2 − x + 1
b) f ( x ) = x − x
009
2
f) f ( x ) = 1− sen 2 x
d) f ( x ) = x − x
a) 2 + ( +` )
c) 2 ⋅ ( +` ) + ( +` )
b) 2 + ( −` )
d) 2 ⋅ ( −` ) ⋅ ( +` )
b) − `
0
c) + `
d) − `
Halla el valor de estas expresiones.
a) 2+` + ( +` ) ⋅ ( +` )
c) ( +` )2 + ( +` )
b) 2−` + ( −` ) ⋅ ( −` )
d) ( −` )2 ⋅ ( +` )
a) + `
011
e) f ( x ) = cos x
3
Determina el valor de las siguientes expresiones.
a) + `
010
c) f ( x ) = x 2 + x − 4
b) + `
c) + `
d) + `
Si lim f ( x ) = −1 y lim g ( x ) = −`, calcula:
x → +`
x → +`
a)
b)
0
lim [ f ( x ) + g ( x )]
c)
lim [ f ( x ) ⋅ g ( x )]
d)
x → +`
x → +`
a)
b)
lim
3
g( x )
lim
3
f(x)
x → +`
x → +`
lim [ f ( x ) + g ( x )] = −`
c)
lim [ f ( x ) ⋅ g ( x )] = + `
d)
x → +`
x → +`
lim
3
g ( x ) = −`
lim
3
f ( x ) = −1
x → +`
x → +`
396
833276 _ 0392-0451.indd 396
21/7/09 15:40:55
7
Solucionario
012
Si lim f ( x ) = +` y lim g ( x ) = −5, halla:
x → −`
a)
b)
lim [ f ( x ) + g ( x )]
c)
lim [ f ( x ) − g ( x )]
d)
x → −`
x → −`
a)
b)
013
b)
g( x )
lim f ( x )g ( x )
x → −`
lim [ f ( x ) + g ( x )] = + `
c)
lim [ f ( x ) − g ( x )] = + `
d) lim f ( x )g ( x ) = 0
x → −`
x → −`
lim x 7
c)
lim x 7
d)
x → +`
x → −`
a)
2x
b)
lim
x → −`
g ( x ) no existe.
x → −`
lim
7
lim
7
x → +`
x → −`
x
e)
x
f)
lim x 7 = + `
c)
lim
7
lim x 7 = −`
d) lim
7
x → +`
x → −`
x → +`
x → −`
lim
x → +`
lim
x → −`
1
x7
1
x7
x = +`
e)
x = −`
f)
calcula estos límites.
a)
b)
lim 7 x
c)
lim 7 x
d)
x → +`
x → −`
a)
b)
c)
015
lim
x → −`
Halla los siguientes límites.
a)
014
x → −`
lim ( 7 )
lim
x → −`
1
x7
1
x7
=0
=0
1
x
e)
x → +`
lim ( 7 )
lim
x → +`
lim 7 x
x → +`
1
x
f)
x → −`
lim 7 x
x → −`
x
lim 7 x = + `
d) lim ( 7 ) = 0
lim 7 x = 0
e)
x → +`
x → −`
1
x → −`
lim 7 x = 1
x → +`
1
x
lim ( 7 ) = + `
f)
x → +`
lim 7 x = 1
x → −`
Halla los límites en el infinito de cada una de estas funciones.
a)
2 x 3
lim
x → +`
x − 1
a)
b)
b)
( 3 x + 1) x 4
lim
x → −`
( x 2 − 6 )( 2 x − 1)3
2 x 3
=8
lim
x → +`
x − 1
lim
x → −`
( 3 x + 1) x 4
2
( x − 6 )( 2 x − 1)3
=
3
8
397
833276 _ 0392-0451.indd 397
21/7/09 15:41:08
límites y continuidad
016
lim
completa
2 x 3 − x + 12
de modo que el resultado sea:
x → +`
a) +`
, escribiendo en su numerador una función
b) 4
c) 0
Respuesta abierta. Por ejemplo:
a)
x4 + 5
lim
x → +`
x → +`
b)
c)
lim
x → +`
x2 + x
3
2 x − x + 12
=0
=4
x2 + 3
2x +1
lim
x → +`
x → +`
b)
c)
2 x2 + x −1
lim
a)
d)
x2 + 3
lim
x → +`
x → −`
x → +`
x2 + 3
x2 + 3
2 x 2 − 12 x + 9
lim
x → +` 3
x2 + 3
1
=
2x +1
2
2 x2 + x − 1
lim
2 x2 + x −1
lim
c)
= +`
d)
x5 + 5 x − 2
lim
2 x2 + x − 1
x → −`
lim
x2 + 3
= +`
2 x 2 − 12 x + 9
x → +` 3
x5 + 5 x − 2
= +`
calcula estos límites.
a)
b)
x +x
x
lim
x → +`
b)
c)
7x + 3x −2
2x
lim
x → +`
a)
019
2 x 3 − x + 12
= +`
resuelve los siguientes límites.
a)
018
2 x − x + 12
8 x3 − 3
b ) lim
017
3
lim
x → +`
d)
2x − 6+ x
2x + 4
lim
x → +`
x +1 + 2x
6x −3
lim
x → −`
x +x
=1
x
c)
7 x + 3x − 2
7
=
x → +`
2x
2
lim
x → +`
d) lim
lim
0
2
x → −`
2x − 6 + x
=1
2x + 4
x2 + 1 + 2 x
1
=
6x −3
2
calcula los siguientes límites.
a)
b)
x 2 − 1 1+ 2 x 2
lim
−
x → +`
x
2 x −1
lim
x → +`
(
x2 − 2 x − x )
c)
d)
lim
( 2 x − 1+ 4 x )
lim
(
x → +`
x → +`
9 x2 + 3 x − 3 x )
398
833276 _ 0392-0451.indd 398
21/7/09 15:41:16
Solucionario
a)
x 2 − 1 1+ 2 x 2
−
lim
2 x −1
x
x → + `
→ ` − `
x 2 − 1 1+ 2 x 2
−
lim
x → + `
2 x −1
x
2
2
= lim ( x − 1)( 2 x − 1) − ( 1 + 2 x ) x =
x → +`
x ( 2 x − 1)
− x2 − 3 x + 1
= lim
2 x2 − x
x → +`
b)
lim
(
x2 − 2 x − x ) → ` − `
lim
(
x 2 − 2 x − x ) = lim
x → +`
x → +`
x2 − 2 x − x2
x → +`
x → +`
c)
(2x −
1+ 4 x
)→ ` −`
lim
(2x −
1+ 4 x
)=
lim
(
9 x2 + 3 x − 3 x ) → ` − `
lim
(
9 x 2 + 3 x − 3 x ) = lim
x → +`
d)
x → +`
x → +`
4 x 2 − 1− 4 x
lim
x → +`
2 x + 1+ 4 x
= +`
9 x2 + 3 x − 9 x2
x → +`
9 x2 + 3 x + 3 x
3x
= lim
x → +`
020
= −1
x2 − 2 x + x
lim
x → +`
1
2
=−
=
x2 − 2 x + x
−2 x
= lim
7
2
9 x + 3x + 3x
=
=
1
2
Sustituye a, b, c y d por números de modo que:
a)
b)
lim
(
x 2 + ax − x ) = 1
lim
(
4 x 2 + bx − 3 − 2 x ) = −
x → +`
x → +`
a)
lim
x → +`
(
c)
x 2 + ax − x ) = lim
x → +`
=
b)
lim
x → +`
(
1
4
lim
(
9 x 2 + 7 − cx ) = 0
lim
(
dx 2 + x − 5 − 2 x ) = + `
x → +`
d)
x → +`
x 2 + ax − x 2
2
x + ax + x
ax
= lim
x → +`
2
x + ax + x
=
a
= 1→ a = 2
2
4 x 2 + bx − 3 − 4 x 2
4 x 2 + bx − 3 − 2 x ) = lim
x → +`
= lim
x → +`
4 x 2 + bx − 3 + 2 x
bx − 3
2
4 x + bx − 3 + 2 x
=
=
b
1
= − → b = −1
4
4
399
833276 _ 0392-0451.indd 399
21/7/09 15:41:19
límites y continuidad
c)
d)
021
9 x 2 + 7 − c2 x 2
lim
(
9 x 2 + 7 − cx ) = lim
lim
(
dx 2 + x − 5 − 2 x ) = lim
x → +`
x → +`
x → +`
9 x2 + 7 + cx
x → +`
dx 2 + x − 5 − 4 x 2
dx 2 + x − 5 + 2 x
= +` → d ≠ 4
calcula los siguientes límites.
a)
2 x −1
1
lim 1 +
x → +`
x
c)
3 x +2
4 x − 1
lim 2 −
x → −`
4 x
b)
6 x +2
3
lim 1−
x → +`
x
d)
x
lim
x → −`
x+3
a)
1
⋅( 2 x −1)
x
b)
= e2
3 6 x +2
lim 1−
→ 1`
x → +`
x
3
⋅( 6 x +2 )
lim −
3 6 x +2
= e x→ +` x
lim 1−
x → +`
x
c)
3 x +1
1 2 x −1
lim 1 +
→ 1`
x → +`
x
lim
1 2 x −1
= e x→ +`
lim 1 +
x → +`
x
1
= e−18 =
0
e18
3 x +2
4 x − 1
lim 2 −
→ 1`
x → −`
4 x
3 x +2
lim 1−
4 x − 1
= e x→ −`
lim 2 −
x → −`
4 x
x
d) lim
x → −`
x+3
x
lim
x → −`
x+3
022
=0→c=3
4 x −1
⋅ ( 3 x +2 )
4 x
lim
3x +2
= e x→ −`
4x
3
= e4 =
4
e3
3 x +1
→ 1`
lim
3 x +1
= e x→ +`
x
x +3
−1⋅( 3 x +1)
lim
−3 ( 3 x +1 )
= e x→ −`
= e−9 =
x +3
1
e
9
Halla estos límites.
1
x 2 + 1 x −1
a) lim
x → +`
x 2
x2 − x + 2
b) lim
x → +`
x2 + 1
x2 −1 2 x
c) lim
x → −`
x 2 + 1
x + 6
x2 − 2 x + 2
d) lim
x → −`
2 x2 + 3 x − 2
x
2
−3 x
400
833276 _ 0392-0451.indd 400
21/7/09 15:41:24
0
7
Solucionario
a)
x 2 + 1 x −1
lim
→ 1`
x → +`
x 2
x 2 +1
lim
x 2 + 1 x −1
= e x→ +`
lim
2
x → +`
x
b)
x2
−1⋅( x −1)
x2 − x + 2
lim
x → +`
x2 + 1
x + 6
→ 1`
x2 − x + 2
lim
x → +`
x 2 + 1
lim
x + 6
x → +`
=e
x 2 − x +2
= e−1 =
x 2 +1
1
lim
⋅( x −1)
x2
= e x → +`
−1⋅( x + 6 )
=e
lim
x → +`
= e0 = 1
− x 2 − 5x + 6
x 2 +1
=
1
e
1
x 2 − 1 2 x
= 10 = 1
c) lim
x → −`
x 2 + 1
x2 − 2 x + 2
d) lim
x → −`
2 x2 + 3 x − 2
023
x
2
−3 x
=0
observa la grafica y calcula:
lim f ( x )
Y
lim f ( x )
x → 0−
x → 0+
f (x)
3
− x + 1 si x ≤ 0
siendo f ( x ) =
x + 2
si x > 0
lim f ( x ) = 1
lim f ( x ) = 2
x →0−
024
X
1
3
x →0+
observa la grafica y halla:
lim f ( x )
x → −2−
lim f ( x )
x → 0−
Y
lim f ( x )
x → −2+
3
lim f ( x )
f (x)
x → 0+
1
lim f ( x ) = −`
x →−2−
lim f ( x ) = + `
x →−2+
lim f ( x ) = 1
x →0−
X
lim f ( x ) = 0
x →0+
401
833276 _ 0392-0451.indd 401
21/7/09 15:41:30
límites y continuidad
025
calcula el límite de la función f ( x ) =
lim f ( x ) =
x →3
x2 − 3
en x = 3 y x = −2.
x +2
0
6
5
lim f ( x ) = −`
1
x →−2−
→ No existe lim f ( x ).
lim f ( x ) = = ` →
x →−2
x →−2
lim f ( x ) = + `
0
x →−2+
0
026
Determina el límite de la función f ( x ) =
1
π
en x =
y x = 0.
sen x
2
lim f ( x ) = 1
x→
π
2
lim f ( x ) = −`
1
x → 0−
→ No existe lim f ( x ).
lim f ( x ) = = ` →
x →0
x →0
lim f ( x ) = + `
0
x → 0+
027
0
resuelve los siguientes límites.
x +1
3x + 3
a) lim
x → −1
2 x2 + 2 x
b) lim
x→0
x +1
0
→
x →−1 3 x + 3
0
a) lim
x2 − 3 x
b) lim
x →0
1
1
x +1
=
lim
= lim
x →−1 3 ( x + 1)
x →−1 3
3
2 x2 + 2 x
2
x − 3x
→
0
0
2 x ( x + 1)
2 ( x + 1)
2
lim
= lim
=−
x →0 x − 3
x →0 x ( x − 3 )
3
0
028
calcula estos límites.
25 − x 2
x −5
a) lim
x →5
a) lim
x →5
lim
x →5
b) lim
2 x 2 − 18
x →3
x2 − 9
25 − x 2
0
→
x −5
0
lim f ( x ) = −`
5+ x
5
( 5 + x )( 5 − x )
x → 5−
i
m
=
=
→
l
=
`
x →5 − 5 − x
lim f ( x ) no existe
0
−( 5 − x )
x → 5+
→ No existe lim f ( x ).
x →5
b) lim
x →3
2 x 2 − 18
2
x −9
→
0
0
lim
x →3
2( x 2 − 9 )
2
x −9
= lim ( 2 x 2 − 9 ) = 0
x →3
402
833276 _ 0392-0451.indd 402
21/7/09 15:41:35
Solucionario
029
Determina si la función f ( x ) =
f ( −2 ) =
f (2) =
030
x +3
x2 − 4
7
es continua en x = −2 y x = 2.
1
→ No existe f ( −2 ) → La función no es continua en x = −2.
0
5
→ No existe f ( 2 ) → La función no es continua en x = 2.
0
Halla si la función f ( x ) = x − 3 es continua en x = −3 y x = 0.
f ( −3 ) = − 6 = 6 → Existe f ( −3 ).
lim x − 3= − 6 = 6 → Existe lim f ( x ).
x →−3
x →−3
f ( −3 ) = lim f ( x ) → La función es continua en x = −3.
x →−3
031
Determina si esta función es continua.
x + 1
si x ≤ −1
f ( x ) = 2
x − 3 si x > −1
• Si x < − 1 → f ( x ) = x + 1 → f ( x ) es continua en (− `, −1).
• Si x > − 1 → f ( x ) = x 2 − 3 → f ( x ) es continua en (−1, + `).
• Si x = − 1 → f ( −1 ) = − 1 + 1 = 0 → Existe f ( −1 ).
→ No existe lim f ( x ).
2
x → −1
lim f ( x ) = lim ( x − 3 ) = −2
+
+
x → −1
x → −1
lim f ( x ) = lim ( x + 1) = 0
x → −1−
2
3
x → −1−
La función no es continua en x = −1, tiene una discontinuidad de salto finito
en este punto, por tanto, f ( x ) es continua en R − {−1}.
032
calcula a para que esta función sea continua en todo R.
x + 1
si x ≤ −2
f ( x ) = x
− x 2 + a si x > −2
• Si x < −2 → f ( x ) =
x +1
→ f ( x ) es continua en ( −`, −2 ).
x
• Si x > −2 → f ( x ) = − x 2 + a → f ( x ) es continua en ( −2 , + ` ).
• Si x = −2 → f ( x ) =
lim f ( x ) = lim
x →−2−
x →−2−
−2 + 1
1
= → Existe f ( −2 ) .
−2
2
x +1
1
=
x
2
lim f ( x ) = lim ( − x 2 + a ) = −4 + a
x →−2+
x →−2+
f ( x ) es continua en x = −2 si:
f ( −2 ) = lim f ( x ) = lim f ( x ) →
−
x →−2
+
x →−2
1
9
= −4 + a → a =
2
2
403
833276 _ 0392-0451.indd 403
21/7/09 15:41:40
límites y continuidad
033
Determina si la función:
0
f ( x ) = sen x + cos x
se anula en algún punto del intervalo (0, 4).
f ( x ) es la suma de funciones continuas en R, por lo tanto es continua en R.
f ( x ) es continua en [0, 4].
f (0) = 1 > 0
f (4) = sen 4 + cos 4 = −1,41 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0, 4) tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 4).
034
Dada la función f ( x ) =
ln x + 2 x
x −2
(0, 1) tal que f ( c ) = 0.
, halla si existe algún punto c en el intervalo
f ( x ) está definida en (0, 2) ∪ (2, + `) → f ( x ) es continua en (0, 2) ∪ (2, + `),
luego, f ( x ) no es continua en [0, 1], ya que no está definida en x = 0.
Para aplicar el teorema de Bolzano podemos considerar el intervalo (0,1; 1).
f ( x ) es continua en [0,1; 1].
f (0,1) = 1,106 > 0
f (1) = −2 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0,1; 1) tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0,1; 1).
Por tanto, también podemos asegurar que existe algún punto c en el intervalo
(0, 1) tal que f ( c ) = 0.
035
Dada la siguiente función:
f ( x ) = sen x + cos x
demuestra que existe un punto c ∈ (0, 4) tal que f ( c ) = −1.
f ( x ) es la suma de funciones continuas en R, por lo tanto es continua en R.
f ( x ) es continua en [0, 4].
f (0) = 1
f (4) = sen 4 + cos 4 = −1,41
Como f ( 0 ) > −1 > f (4) podemos aplicar el teorema de los valores
intermedios → Existe c ∈ (0, 4) tal que f ( c ) = −1.
036
ln x + 2 x
, demuestra que alcanza un máximo
x −2
y un mínimo absolutos en un intervalo.
Dada la función f ( x ) =
f ( x ) es continua en (0, 2) ∪ (2, + `), por tanto, f ( x ) es continua en [0,1; 1].
Entonces, por el teorema de Weierstrass, existe al menos un punto donde
la función alcanza su valor máximo absoluto y otro donde toma su valor mínimo
absoluto.
404
833276 _ 0392-0451.indd 404
21/7/09 15:41:41
0
Solucionario
037
Determina el término general de las siguientes sucesiones, y calcula su límite.
a) 1,
1 1 1 1 1
, , ,
,
,…
2 4 8 16 32
d) −
b) 0 ,
1 2 3 4 5
, , , , ,…
2 3 4 5 6
e) 64 , 32 , 16 , 8 , 4 , 2 , …
c) 1,
5 7 9 11 13
, , ,
,
,…
3 3 3 3 3
2 4
8 16
32
, ,−
,
,−
,…
3 9
27 81
243
1
a) an =
1
lim
n−1
n→ `
b) an =
n −1
n
n→ `
c) an =
3 + 2 ( n − 1)
2n + 1
=
3
3
n→ `
d) an =
2
( −2 )n
2
n−1
n −1
=1
n
lim
2n + 1
= +`
3
( −2 )n
n→ `
3
=0
lim
lim
n
1 n−1
26
= 27−n
e) an = 64 ⋅ =
2
n−1
2
038
7
3n
no existe.
lim 27−n = 0
n→ `
Halla el límite de estas sucesiones expresadas por su término general.
f ) fn = ( n + 3 )( 2 n − 3 )
a) an = 3 n + 1
b) bn =
5
n +1
g) gn = 2 n−1
c) cn = n2 − 5 n + 6
3 n
h) hn =
5
d) dn = 3 − n + n2 − n3
i) in = 3 3 n−1
2
2
e) en = 3 −
n−4
2
j) kn =
n2 + 3 n − 2
n
a) lim ( 3 n + 1) = + `
f ) lim [( n + 3 )( 2 n − 3 )] = + `
5
b) lim
=0
n→ ` n + 1
g) lim 2n−1 = + `
n→ `
n→ `
n→ `
2
c) lim ( n − 5 n + 6 ) = + `
3 n
h) lim = 0
n→ `
5
d) lim ( 3 − n + n2 − n3 ) = −`
i)
n−4
e) lim 3 −
n→ `
2
j) lim
2
n→ `
2
n→ `
= −`
lim 3 3 n−1 = 30 = 1
n→ `
n→ `
n2 + 3 n − 2
= +`
n
405
833276 _ 0392-0451.indd 405
21/7/09 15:41:50
límites y continuidad
039
calcula los siguientes límites de sucesiones.
a) lim ( − n3 + 8 n2 − n + 8 )
d) lim
n →`
b) lim
3
n →`
c) lim
n →`
n →`
2 n3 + 1
e) lim
n2 − 3 n − 2
f ) lim
n →`
3 n2 − 8 n + 16
35
6n − 2
3
3n − 7n + 1
5 − 2 n + 3 n2 − n3
n →`
n→ `
n→ `
c) lim
n→ `
040
3
0
3 n2 − 8 n + 16
= +`
d) lim
n→ `
35
a) lim ( − n3 + 8 n2 − n + 8 ) = −`
b) lim
2 n2 − 5 n − 4
2 n3 + 1 = + `
e) lim
n2 − 3 n − 2 = + `
f ) lim
n→ `
n→ `
6n − 2
3 n3 − 7 n + 1
=0
5 − 2 n + 3 n2 − n3
2 n2 − 5 n − 4
= −`
calcular razonadamente el límite de la sucesión:
( n − 2 )2
( n + 1)3 − ( n − 1)3
(Aragón. Septiembre 2005. Opción B. Cuestión 3)
lim
n→ `
( n − 2 )2
3
= lim
3
( n + 1) − ( n − 1)
n→ `
= lim
n→ `
n2 − 4 n + 4
3
2
n + 3 n + 3 n + 1− n3 + 3 n2 − 3 n + 1
n2 − 4 n + 4
6 n2 + 2
=
=
1
6
0
041
Determina los límites de estas sucesiones.
4 n2 − 1
6 n
a) lim
⋅
3
n →`
5n
n + 1
2 n2 + 3
6 n − n2
c) lim
+
n →`
5n
3n
n2 + 5
5 n3
b) lim
:
n →`
1− 2 n n2 + 12
3n + 2
d) lim
n →`
6 n2
( 8 n − 1)
4 n2 − 1
6 n
24 n2 − 6
= lim
a) lim
⋅
=0
n→ `
5n
n3 + 1 n→ ` 5 n3 + 5
n2 + 5
5 n3
b) lim
:
n→ `
1− 2 n n2 + 12
2
2
= lim ( n + 5 )( n + 12 ) =
n→ `
5 n3 ( 1− 2 n )
= lim
n→ `
n4 + 17 n2 + 60
5 n3 − 10 n4
=−
1
10
406
833276 _ 0392-0451.indd 406
21/7/09 15:41:57
Solucionario
2 n2 + 3
6 n − n2
+
c) lim
n→ `
3n
5n
2
2
= lim 6 n + 9 + 30 n − 5 n =
n→ `
15 n
= lim
n→ `
3n + 2
d) lim
2
n→ `
6n
042
7
n2 + 30 n + 9
= +`
15 n
24 n2 + 13 n − 2
( 8 n − 1) = lim
=4
n→ `
6 n2
Dejamos caer una pelota desde una altura de 4 metros y, tras cada rebote,
la altura alcanzada se reduce a la mitad de la altura anterior.
¿Qué altura alcanzará la pelota después de cada uno de los cinco primeros rebotes?
¿Y tras el vigésimo rebote? ¿Y tras el rebote n-ésimo? Si an denota la altura alcanzada
tras el n-ésimo rebote, obtén una cota superior y otra inferior de esta sucesión.
calcula lim an .
n →`
(Galicia. Septiembre 2004. Bloque 4. Pregunta 1)
a1 = 2 m
a2 = 1 m
a3 =
1
m
2
a4 =
a5 =
1
m
8
1 n−1
2
1
=
= 2− ( n−2 )
a n = 2 ⋅ =
2
n−1
n−2
2
2
1 19
2
1
=
m
a 20 = 2 ⋅ =
2
19
.144
262
2
1
2
1
m
4
1 n−1
2
1
=
= 2− ( n−2 )
a n = 2 ⋅ =
2
n−1
n−2
2
2
19
2
1
=
=
m
19
.144
262
2
Una cota superior de la sucesión es 4 y una inferior es 0.
lim 2− ( n−2 ) = 0
n→ `
043
observa las gráficas de estas funciones, y calcula los siguientes límites.
Y
Y
f (x)
X
a)
b)
lim f ( x )
c)
lim f ( x )
d)
x → +`
x → −`
a)
b)
g( x )
lim g ( x )
x → +`
lim g ( x )
x → −`
lim f ( x ) = + `
c)
lim f ( x ) = −`
d) lim g ( x ) = + `
x → +`
x → −`
X
lim g ( x ) = −`
x → +`
x → −`
407
833276 _ 0392-0451.indd 407
21/7/09 15:42:02
límites y continuidad
044
Halla estos límites de funciones.
a)
b)
c)
d)
lim x 5
e)
lim x 5
f)
x → +`
x → −`
lim
3
lim
3
x → +`
x → −`
a)
b)
x2
g)
x2
h)
1
lim
x → +`
x4
i)
1 x
lim
x → +`
3
j)
1 x
lim
x → −`
3
1
lim
x → −`
x4
lim 5 x
k)
lim 5 x
l)
x → +`
x → −`
lim x 5 = + `
g)
lim x 5 = −`
h)
x → +`
x → −`
lim 4 x
2
lim 4 x
2
x → +`
x → −`
lim 5 x = + `
x → +`
lim 5 x = 0
x → −`
lim
3
x 2 = +`
i)
1 x
lim = 0
x → +`
3
d) lim
3
x 2 = +`
j)
1 x
lim = + `
x → −`
3
c)
x → +`
x → −`
e)
f)
1
lim
x → +`
x
4
1
lim
x → −`
x4
=0
k)
=0
l)
0
2
lim 4 x = + `
x → +`
2
lim 4 x = + `
x → −`
0
045
calcula los siguientes límites de funciones.
a)
b)
c)
d)
e)
f)
lim
x2 + 1
x −3
g)
lim
x2 + 1
x −3
h)
x → +`
x → −`
lim
x → +`
lim
x → −`
lim
x → +`
lim
x → −`
x2 + 1
3 x2
x2 + 1
3 x2
1− x 6
2
3 x + 2 x −1
1− x 6
3 x2 + 2 x −1
i)
j)
k)
l)
lim
x → +`
lim
x → −`
lim
x → +`
lim
x → −`
1− x 4
−x 4 + 2 x2 − 5
1− x 4
−x 4 + 2 x2 − 5
x3 − 3 x2 − 5
x2 − 2 x + 3
x3 − 3 x2 − 5
lim
16
x −2
lim
16
x −2
x → +`
x → −`
0
x2 − 2 x + 3
408
833276 _ 0392-0451.indd 408
21/7/09 15:42:14
Solucionario
a)
b)
c)
lim
x2 + 1
= +`
x −3
g)
lim
x2 + 1
= −`
x −3
h)
x → +`
x → −`
x2 + 1
lim
3 x2
x → +`
x2 + 1
d) lim
3 x2
x → −`
e)
f)
046
=
1
3
i)
=
1
3
j)
1− x 6
lim
x → +`
3 x2 + 2 x − 1
1− x 6
lim
x → −`
2
3x + 2 x −1
= −`
k)
= −`
l)
Se considera la función f ( x ) =
x
x2 + 1
1− x 4
lim
−x 4 + 2 x2 − 5
x → +`
1− x 4
lim
−x 4 + 2 x2 − 5
x → −`
x2 − 2 x + 3
lim
x3 − 3 x2 − 5
x → +`
x2 − 2 x + 3
lim
x3 − 3 x2 − 5
x → −`
lim
16
=0
x −2
lim
16
=0
x −2
x → +`
x → −`
7
=1
=1
=0
=0
. calcula lim f ( x ) y lim f ( x ).
x → +`
x → −`
(Baleares. Septiembre 2008. Opción A. Cuestión 3)
x
lim
x → +`
x2 + 1
=0
lim
x
x → −`
x2 + 1
=0
1
047
x
Halla el límite: lim
x →`
x + 1 − x −1
(Navarra. Septiembre 2005. Grupo 2. Opción C)
1
lim
x → +`
048
x(
x +1 −
x −1 )
x ( x + 1) +
= lim
x → +`
x ( x − 1)
( x2 + x ) − ( x2 − x )
=1
Determina los siguientes límites de funciones.
a)
b)
lim ( x 3 − 12 x )
c)
lim ( x − 0 , 001x 2 )
d)
x → +`
x → +`
a)
b)
lim 0 , 62 x −1
x → +`
lim ( 2 x − 3 ) x
x → +`
lim ( x 3 − 12 x ) = + `
c)
lim ( x − 0 , 001x 2 ) = −`
d)
x → +`
x → +`
lim 0 , 62 x −1 = 0
x → +`
lim ( 2 x − 3 ) x = + `
x → +`
409
833276 _ 0392-0451.indd 409
21/7/09 15:42:23
límites y continuidad
049
resuelve los siguientes límites.
a)
b)
lim
(x−
x2 − 4 x
)
c)
1− x
2
lim 1 +
x → −`
x
lim
(x+
x2 − 4 x
)
d)
1− x
2
lim 1−
x → −`
x
x → −`
x → −`
a)
b)
lim
(x−
x2 − 4 x
) = −`
lim
(x+
x2 − 4 x
) → −` + `
(x+
x→ −
x2 − 4 x
) = x →lim−
x → −`
x → −`
lim
c)
`
0
x2 − x2 + 4 x
`
x−
2
2
x −4x
= lim
x → −`
4x
x−
x2 − 4 x
=2
1− x
2
lim 1 +
→ 1`
x → −`
x
2−2 x
1− x
lim 1+ −1⋅( 1− x )
lim
1
2
= e x → −` x
= e−2 =
lim 1 + = e x→ −` x
x → −`
x
e2
0
1− x
2
d) lim 1− → 1`
x → −`
x
2
−2+2 x
1− x
lim 1− −1⋅( 1− x )
lim
2
= e x → −`
x
= e2
lim 1− = e x→ −` x
x → −`
x
050
calcula el límite: lim
x → +`
x2 − 2 x − ( x − 2)
x −2
(La Rioja. Septiembre 2005. Propuesta A. Ejercicio 5)
lim
x → +`
lim
x → +`
051
x2 − 2 x − ( x − 2 )
→`−`
x −2
x2 − 2 x − ( x − 2 )
x 2 − 2 x − ( x − 2 )2
= lim
=
x → +`
x −2
( x − 2 )( x 2 − 2 x + ( x − 2 ) )
2x − 4
=0
= lim
2
x → +`
( x − 2 ) x − 2 x + ( x − 2 )2
calcula el límite: lim
x → +`
0
x + 1 − x −1
x +2 − x −2
(Navarra. Junio 2008. Grupo 1. Opción C)
lim
x → +`
x +1 −
x −1
x+2 −
x −2
→`−`
410
833276 _ 0392-0451.indd 410
21/7/09 15:42:28
Solucionario
lim
x → +`
x +1 −
x −1
x+2 −
x −2
= lim
x → +`
= lim
x → +`
052
053
( x + 1− x + 1)(
2( x + 2 +
)
x −1 )
=
)
)
x −1
x −2
x +1 +
x −2
4( x + 1 +
x2 + 1
2 x4 + 3x
Halla el límite: lim
x −
x → +`
x2 −1
→ ` − `
x2 + 1 2 x 4 + 3 x
lim
−
x → +`
x
x2 − 1
4
5
2
= lim x − 1− 2 x − 3 x = −`
x → +`
x ( x 2 − 1)
x3 + 2 x2 − 3
2 x2 − x
Determina el límite: lim
+
2
x → −`
x −1
x −2
x3 + 2 x2 − 3
2 x2 − x
+
lim
2
x → −`
x −1
x −2
→ −` + `
x3 + 2 x2 − 3
2 x2 − x
lim
+
x → −`
x −1
x2 − 2
=
x → −`
calcular lim
x → +`
(
x 4 + x3 − 2 x2 − 3 x + 3 + 2 x 4 − x3 − 4 x2 + 2 x
( x 2 − 2 )( x − 1)
x → −`
= lim
=
1
2
x2 + 1 2 x 4 + 3 x
lim
−
x → +`
x
x2 − 1
= lim
054
x+2 +
( x + 2 − x + 2 )(
7
3 x 4 − 6 x2 − x + 3
x3 − x2 − 2 x + 2
=
= −`
4 x 2 + 1 − 4 x 2 − 3 x + 2 ).
(Aragón. Septiembre 2008. Bloque 3. Opción A)
lim
(
4 x2 + 1 − 4 x2 − 3 x + 2 ) → ` − `
lim
(
4 x 2 + 1 − 4 x 2 − 3 x + 2 ) = lim
x → +`
x → +`
x → +`
= lim
x → +`
4 x 2 + 1− ( 4 x 2 − 3 x + 2 )
4 x2 + 1 + 4 x2 − 3 x + 2
3x −1
2
4x +1+
2
4 x − 3x + 2
=
=
3
4
411
833276 _ 0392-0451.indd 411
21/7/09 15:42:31
límites y continuidad
055
calcula el límite lim
x → +`
(
x 2 + 2 x − x ).
0
(Navarra. Junio 2004. Grupo 2. Opción C)
lim
(
x2 + 2 x − x ) → ` − `
lim
(
x 2 + 2 x − x ) = lim
x → +`
x → +`
056
x → +`
x+
calcula el límite: lim
x → +`
x2 + 2 x − x2
2
x + 2x + x
2x
= lim
x → +`
2
x + 2x + x
=1
x − x
(Navarra. Septiembre 2004. Grupo 2. Opción C)
lim
(
x+
x −
x
)→ ` −`
lim
(
x+
x −
x
)=
x → +`
x → +`
057
0
x+
lim
x → +`
x+
x −x
x +
x
x
= lim
x → +`
x+
x +
=
x
1
2
x
2
calcula: lim 1 +
x → +`
x
(Asturias. Septiembre 2006. Bloque 5)
058
2
2x
x
lim 1+ −1⋅ x
lim
2
lim 1 + = e x → +` x = e x→ +` x = e 2
x → +`
x
x
2
lim 1 + → 1`
x → +`
x
0
Halla los siguientes límites.
a)
2 + 5x
lim
x → +`
1+ 5 x
2 x −12
2 + 5x
a) lim
1+ 5 x
x → +`
2 + 5x
lim
x → +`
1+ 5 x
b)
0
2 x −12
→ 1`
2+
+5 x
−1⋅( 2 x −12 )
lim
2 x −12
x→ +
= e ` 1+ 5 x
=
lim
( 2+5 x −1−5 x )( 2 x −12 )
1+ 5 x
= e x → +`
b)
2 x +2
x2 + 2 x − 3
lim
x → +`
x2 + 3 x
x2 + 2 x − 3
lim
x → +`
x 2 + 3 x
2 x +2
→ 1`
x2 + 2 x − 3
lim
x → +`
x2 + 3 x
lim
2 x +2
x → +`
=e
x 2 +2 x −3
lim
= e x→ +`
x 2 +3 x
−1 ⋅ ( 2 x +2 )
( x 2 +2 x −3− x 2 −3 x )( 2 x +2 )
x 2 +3 x
lim
= e x → +`
lim
= e x→ +`
2 x −12
2
1+ 5 x
= e5 =
5
e2
=
−2 x 2 −8 x −6
x 2 +3 x
= e−2 =
1
e2
412
833276 _ 0392-0451.indd 412
21/7/09 15:42:35
Solucionario
059
x +5
calcular lim
x → +`
x −1
7
x2
x +3
.
(Aragón. Septiembre 2006. Opción A. Cuestión 3)
x2
x+5
lim
x → +`
x −1
x +3
→ 1`
x+5
lim
x → +`
x −1
lim
x +3
x→ +
= e `
x +5 x 2
−1 ⋅
x −1 x +3
x2
lim
= e x → +` x
060
=
2
=e
( x +5− x +1 )⋅ x 2
( x −1)( x +3 )
=
6 x2
= e6
+2 x −3
3 x + 1 x
calcula el límite: lim
x → +`
3 x − 1
(Navarra. Septiembre 2004. Grupo 2. Opción C)
1
2
3 x + 1 x
→ 1`
lim
x → +`
3 x − 1
3 x +1
lim
−1 ⋅ x
lim
3 x + 1 x
= e x→ +` 3 x −1 = e x→ +`
lim
x → +` 3 x − 1
e2
lim
x → +`
061
2x − 8
calcula: lim
x → +`
2 x +1
( 3 x +1−3 x +1) ⋅ x
lim
2x
2
= e x → +` 3 x −1 = e 3
3 x −1
(Asturias. Junio 2007. Bloque 4)
2x − 8
lim
x → +`
2 x +1
062
3
x
= lim 2 − 2
x → +` 2 x +1
2 x +1
2
= lim 1 − 2
x → +` 2
2x
= 1
2
Expresa las funciones siguientes como funciones definidas a trozos y, después,
halla sus límites cuando x tiende a −` y a +`.
a) f ( x ) = x + 2 − x − 2
b) f ( x ) = x − 3 − 2 x
2
1
e2
c) f ( x ) =
2x +3
x −2
d) f ( x ) =
x −3
1− x
− x − 2 − ( − x + 2 ) si x < −2
−4 si x < −2
a) f ( x ) = x + 2 − ( − x + 2 ) si − 2 ≤ x < 2 → f ( x ) = 2 x si − 2 ≤ x < 2
si x ≥ 2
si x ≥ 2
4
x + 2 − ( x − 2 )
lim f ( x ) = lim ( −4 ) = −4
x → −`
x → −`
lim f ( x ) = lim 4 = 4
x → +`
x → +`
413
833276 _ 0392-0451.indd 413
21/7/09 15:42:40
límites y continuidad
3
x − ( 3 − 2 x )
si x <
2
b) f ( x ) =
3
x − ( −3 + 2 x ) si x ≥
2
3
3 x − 3 si x <
2
→ f(x)=
3
− x + 3 si x ≥
2
lim f ( x ) = lim ( 3 x − 3 ) = −`
x → −`
lim f ( x ) = lim
x → −`
x → −`
x − 3
−
1− x
x −3
d) f ( x ) =
1− x
x − 3
−
1− x
si x < −
si −
x → +`
x → +`
3
2
3
≤ x <2
2
si x > 2
2x + 3
=2
x −2
lim f ( x ) = lim
x → +`
x → +`
2x + 3
=2
x −2
si x < 1
si 1 < x < 3
sii x ≥ 3
x −3
lim f ( x ) = lim −
x → −`
x → −`
1− x
063
lim f ( x ) = lim ( − x + 3 ) = −`
x → −`
2 x + 3
− 2
x
2x + 3
c) f ( x ) = −
x −2
2
+3
x
x − 2
0
x −3
lim f ( x ) = lim −
x → +`
x → +`
1− x
= 1
= 1
0
la siguiente representación es la gráfica de la función f ( x ).
Y
f (x)
2
2
X
Da un valor aproximado a estos límites.
a) lim f ( x )
d) lim f ( x )
b) lim f ( x )
e)
c) lim f ( x )
f)
x →1
x→0
x →2
0
x →3
lim f ( x )
x → +`
lim f ( x )
x → −`
a) lim f ( x ) = −0 , 9
c) lim f ( x ) = 4
e)
b) lim f ( x ) = 0
d) lim f ( x ) = −1
f)
x →1
x →0
x →2
x →3
lim f ( x ) = −`
x → +`
lim f ( x ) = −`
x → −`
414
833276 _ 0392-0451.indd 414
21/7/09 15:42:47
Solucionario
064
Esta es la gráfica de la función g ( x ).
Y
2
`
1
`
`
7
X
1
g( x )
Da un valor aproximado de los siguientes límites.
a)
lim g ( x )
b) lim g ( x )
x→0
a)
c) lim g ( x )
e)
d) lim g ( x )
f)
x →1
x → −3
x →2
lim g ( x ) = 0 , 7
x → −3
x →0
Si f ( x ) =
3x
x2 + 1
lim g ( x )
x → −`
c) lim g ( x ) = −2 , 9
e)
d) lim g ( x ) = 0
f)
x →1
b) lim g ( x ) = 0
065
lim g ( x )
x → +`
x →2
lim g ( x ) = + `
x → +`
lim g ( x ) = 0
x → −`
, calcula estos límites.
a) lim f ( x )
b) lim f ( x )
x →3
a) lim f ( x ) =
x →3
b) lim f ( x ) =
x →−1
x → −1
9
10
−3
2
=
c) lim f ( x )
x→0
9 10
10
=−
3 2
2
c) lim f ( x ) = 0
x →0
066
Dada f ( x ) = 2 ln x, determina:
a) lim f ( x )
x→e
b)
lim f ( x )
x → −5
c) lim f ( x )
x→0
a) lim f ( x ) = 21 = 2
x →e
b) lim f ( x ) no existe porque no podemos calcular logaritmos de números
x → −5
negativos.
c) lim f ( x ) no existe ya que lim f ( x ) = 0 y lim f ( x ) no existe.
x →0
x →0+
x →0−
415
833276 _ 0392-0451.indd 415
21/7/09 15:42:56
límites y continuidad
067
Si tenemos la función f ( x ) =
6 x − 12
2
x −3x − 4
cuando x tienda a 0, −1, 1 y 4?
6 x − 12
lim
x →0
x − 3x − 4
6 x − 12
x → −1
x2 − 3 x − 4
6 x − 12
lim
x →1
=3
2
lim
x2 − 3 x − 4
, ¿cuáles serán sus límites
=
lim f ( x ) = −`
−
−18
→ No existe lim f ( x ).
= ` → x → −1
x → −1
lim f ( x ) = + `
0
+
x → −1
=1
0
lim f ( x ) = −`
−
6 x − 12
12
→ No existe lim f ( x ).
lim
=
= ` → x→4
2
x →4
x→4 x − 3 x − 4
lim f ( x ) = + `
0
+
x→4
068
Siendo la función g ( x ) = log ( x 2 − 4 ), determina sus límites cuando x
tienda a 5, −5, −2 y 1.
lim log( x 2 − 4 ) = log 21 = 1, 32
x →5
lim log( x 2 − 4 ) = log 0 →
x → −2
lim log( x 2 − 4 ) = log 21 = 1, 32
x → −5
lim g ( x ) = −`
→ No existe lim g ( x ).
x →−2
lim g ( x ) no existe
+
x → −2
x → −2−
0
lim log( x 2 − 4 ) no existe.
x →1
069
Si sabemos que lim m ( x ) = 4 , lim n ( x ) = 0 y lim p ( x ) = +`, calcula,
x →5
x →5
x →5
si es posible, el límite cuando x tiende a 5 de las funciones.
n( x )
m( x )
p( x )
a) m ( x ) + n ( x ) + p ( x )
d)
b) m ( x ) ⋅ n ( x ) − p ( x )
m( x )
e)
n( x )
m( x )
n( x )
h)
p( x )
c) m ( x ) ⋅ p ( x )
f ) n( x ) ⋅ p( x )
i)
g)
n( x )
( m( x ) )
p( x )
j)
( m( x ) )
k)
( n( x ) )
l)
( p( x ) )
p( x )
n( x )
a) lim [ m ( x ) + n ( x ) + p ( x )] = + `
x →5
b) lim [ m ( x ) ⋅ n ( x ) − p ( x )] = −`
x →5
c) lim [ m ( x ) ⋅ p ( x )] = + `
x →5
n( x )
d) lim
x →5 m ( x )
=0
m( x )
4
=
e) lim
→ No se puede calcular el límite.
x →5 n ( x )
0
416
833276 _ 0392-0451.indd 416
21/7/09 15:43:05
Solucionario
7
f ) lim [ n ( x ) ⋅ p ( x )] → 0 ⋅ + ` Indeterminación
x →5
p( x )
g) lim
x →5 m ( x )
= +`
j ) lim [( m ( x )) p ( x ) ] = + `
n( x )
h) lim
x →5 p ( x )
=0
k) lim [( n ( x )) p ( x ) ] = 0
i ) lim [( m ( x ))
n( x )
x →5
070
Si h ( x ) =
lim
x →6
x →5
x →5
0
]= 4 =1
l ) lim [ ( p ( x ))n ( x ) ] → + ` 0 Indeterminación
x →5
1
, calcula su límite en los puntos 6, −5, 1 y 0.
ln x
1
1
=
= 0 , 56
ln x
ln 6
lim
x →−5
1
no existe.
ln x
lim h ( x ) = −`
−
1
1
→ No existe lim h ( x ).
= = ` → x →1
x →1 ln x
x →1
lim h ( x ) = + `
0
x →1+
lim
lim
x →0
071
lim h ( x ) no existe
−
1
1
→ No existe lim h ( x ).
→ x →0
=
x →0
lim h ( x ) = 0
ln x
ln 0
x → 0+
observa la gráfica y determina los siguientes límites.
a)
Y
lim f ( x )
x → −`
X
2
x)
lim f ( x )
x → −2−
f (x)
2
lim f ( x )
x → −2+
lim f ( x )
x → +`
x)
b)
Y
lim g ( x )
g( x )
x)
x → −`
2
lim g ( x )
1
X
x → −2 −
lim g ( x )
x → 1−
c)
Y
lim h ( x )
x → −`
h( x )
2
2
lim g ( x )
x → +`
lim g ( x )
x → −2 +
lim g ( x )
x → 1+
d)
Y
lim m ( x )
m( x )
lim h ( x )
X
x → −2−
lim h ( x )
x → −2+
lim h ( x )
x → +`
x → −`
lim m ( x )
x → 1−
1
1
X
lim m ( x )
x → 1+
lim m ( x )
x → +`
417
833276 _ 0392-0451.indd 417
21/7/09 15:43:12
límites y continuidad
a)
lim f ( x ) = 1
lim f ( x ) = + `
x → −`
lim f ( x ) = −`
lim f ( x ) = 1
x → +`
x → −2−
b)
lim g ( x ) = 0
lim g ( x ) = + `
x → −`
lim g ( x ) = −`
lim g ( x ) = + `
lim h ( x ) = −`
lim g ( x ) = 0
x → +`
lim h ( x ) = + `
x → −`
x →−2+
lim h ( x ) = −`
lim ( x ) = + `
x → +`
d) lim m ( x ) = −`
lim m ( x ) = + `
x → −`
x →1+
lim m ( x ) = + `
lim m ( x ) = + `
x → +`
x →1−
observa la gráfica de la función f ( x ), y calcula los límites.
Y
a) lim f ( x )
f (x)
x →1
b)
c)
lim f ( x )
x → 4−
1
1
lim f ( x )
X
x → 4+
b) lim f ( x ) = 3
a) lim f ( x ) = −1
c)
x →4−
x →1
073
x →1+
x →1−
x → −2−
072
lim g ( x ) = −`
x → −2+
x → −2−
c)
0
x → −2+
lim f ( x ) = 5
x →4+
Esta es la gráfica de la función p ( x ).
Y
0
1
1
X
p( x )
0
Determina los siguientes límites.
a) lim p ( x )
x→0
b)
lim p ( x )
x → −1−
a) lim p ( x ) = 0
x →0
b)
lim p ( x ) = −`
x → −1−
c)
lim p ( x )
x → −1+
d) lim p ( x )
x → 1−
c)
lim p ( x ) = + `
x → −1+
d) lim p ( x ) = + `
x →1−
e) lim p ( x )
x → 1+
f)
lim p ( x )
x → +`
e) lim p ( x ) = −`
x →1+
f)
lim p ( x ) = + `
x → +`
418
833276 _ 0392-0451.indd 418
21/7/09 15:43:20
`
`
Solucionario
074
resuelve estos límites.
lim
x2 − 2 x + 1
x −3
b) lim
x2 − 2 x + 1
x −3
a)
x → 2−
−
x →3
c)
x2 − 2 x + 1
= −`
x −3
c) lim
2
x −2x +1
d) lim
−
x →2
lim
x → 2+
x2 + x − 6
3
x − x 2 − 8 x + 12
x2 + x − 6
3
2
x − x − 8 x + 12
x2 − 3 x + 2
calcula lim
x → 1−
x2 − 2 x + 1
lim
x2 − 2 x + 1
= −1
x −3
lim
x2 − 2 x + 1
= +`
x −3
+
x →2
+
x →3
= −`
x2 − 9
x → 3−
x 3 − x 2 − 8 x + 12
x → 2+
b) lim
x →3
x2 + x − 6
lim
x2 − 2 x + 1
= −1
x −3
−
x2 −9
x →3
a) lim
x →2
x2 −2 x + 1
+
x 3 − x 2 − 8 x + 12
−
x2 − 2 x + 1
x −3
lim
x2 + x − 6
x → 2−
lim
x →3
x −9
x →3
x2 − 2 x + 1
x −3
+
2
−
lim
x → 2+
x2 −2 x + 1
lim
d) lim
075
7
lim
x → 3+
x2 − 2 x + 1
x2 − 9
( x − 2 )( x + 3 )
= lim
( x − 2 )2 ( x + 3 )
x → 2−
= +`
= lim
x → 2−
1
= −`
x −2
1
= +`
x −2
= lim
x → 2+
.
(Navarra. Junio 2001. Opción D. Pregunta 1)
lim
x2 − 3 x + 2
−
x →1
076
2
x −2x +1
= lim
−
x →1
( x − 2 )( x − 1)
2
( x − 1)
= lim
−
x →1
x −2
= +`
x −1
Determina los límites siguientes y, en caso de resultar infinito,
halla los límites laterales.
a) lim
x →2
b)
lim
x2 − x − 2
2
2x −3x −2
x3 + 5 x2 + 6 x
x → −2
c) lim
x →1
x 3 + x 2 − 8 x − 12
3 x 3 − 18 x 2 + 27 x
5 x 2 − 20 x + 15
x2 − x − 2
lim
x → −1
lim
x3 + 5 x2 + 6 x
x → −3
lim
x →3
2 x2 − 3 x − 2
x 3 + x 2 − 8 x − 12
3 x 3 − 18 x 2 + 27 x
5 x 2 − 20 x + 15
419
833276 _ 0392-0451.indd 419
21/7/09 15:43:24
límites y continuidad
x2 − x − 2
a) lim
x →2
2
2x − 3x − 2
x2 − x − 2
lim
x → −1
2 x2 − 3 x − 2
x → −2
3
lim
3
x + x − 8 x − 12
x3 + 5 x2 + 6 x
3
2
x + x − 8 x − 12
x 3 + x 2 − 8 x − 12
2
5 x − 20 x + 15
lim
x →1−
= +`
lim
x →1+
5 x 2 − 20 x + 15
3 x 3 − 18 x 2 + 27x
5 x 2 − 20 x + 15
5 x − 20 x + 15
= lim
x →3
Se considera la función f ( x ) =
2
( x − 3 )( x + 2 )
=
−2
=`
0
= lim
x ( x + 3)
= −`
( x − 3 )( x + 2 )
= lim
x ( x + 3)
= +`
( x − 3 )( x + 2 )
x → −2−
x → −2+
12
=`
0
3 x 3 − 18 x 2 + 27x
2
x ( x + 2 )(( x + 3 )
=0
=
3 x 3 − 18 x 2 + 27x
x →3
077
2
3 x 3 − 18 x 2 + 27x
x →1
lim
3
x + 5 x2 + 6 x
x →−3
5 x 2 − 20 x + 15
2
3
lim
lim
x → −2
x + 5x + 6 x
x → −2+
c) lim
= lim
x + x − 8 x − 12
lim
x →1−
=0
2
x → −2−
3 x 3 − 18 x 2 + 27x
x →2
x3 + 5 x2 + 6 x
b) lim
x +1
3
( x − 2 )( x + 1)
= lim
=
x →2 2 x + 1
5
( x − 2 )( 2 x + 1)
= lim
= +`
lim
x →1+
3 x 3 − 18 x 2 + 27x
5 x 2 − 20 x + 15
= −`
0
= −`
3 x ( x − 3)
3 x ( x − 3 )2
= lim
=0
x
→
3
5( x − 1)( x − 3 )
5( x − 1)
x2
. calcula lim f ( x ), lim f ( x ) y lim f ( x ).
x →1
x → +`
x → −`
x −1
(Baleares. Septiembre 2008. Opción B. Cuestión 3)
x2
= −`
1
x
= = ` → x →1 x − 1
lim
→ No existe lim f ( x ).
2
x →1 x − 1
x →1
0
x
= + `
lim
x →1+ x − 1
2
lim
x → +`
078
lim
−
x2
= +`
x −1
lim
x → −`
x2
= −`
x −1
obtén los resultados de estos límites.
x
a) lim
x→0 3
x
3
x
b) lim
x→0
x
c) lim
x →2 3
d) lim
x →2
x −2
2
x −3x + 2
x −2
x−4
e) lim
x→4
f ) lim
x →9
x −2
x−4
x −9
x −3
420
833276 _ 0392-0451.indd 420
21/7/09 15:43:31
Solucionario
x
a) lim
x →0 3
b) lim
3
x →0
= lim
6
x →0
x=0
no existte
x
1
1
1
x →0
x
= lim
= =`→
→ No existe lim 6 .
6
x →0
x →0
0
1
x
x
x
= + `
lim
x → 0+ 6 x
lim
−
x −2
c) lim
x →2 3
x →2 3
( x − 2 )( x − 1)
e) lim
x −2
x−4
= lim
= lim
x → 4 ( x − 4 )( x + 2 )
x→4
x−4
x −9
f ) lim
x →9
Si f ( x ) =
x −3
= lim
x →9
x3 + 7 x2
a) lim f ( x )
lim f ( x )
lim f ( x )
x 3 + 7x 2
x →3
=
x 3 + 11x 2 + 31x + 21
3
x +7x
x → −7
2
= lim
x → −7
x → −7
d) lim
e)
f)
x3 + 7 x2
x 3 + 11x 2 + 31x + 21
lim
x → +`
lim
2
x + 11x + 31x + 21
x →0
x → −`
=0
1
4
f)
x 3 + 7x 2
x 3 + 7x 2
x 3 + 11x 2 + 31x + 21
x 3 + 7x 2
lim f ( x )
x → −`
( x + 1)( x + 3 )( x + 7 )
x2 ( x + 7 )
( x + 1)( x + 3 )
x
2
=
=
24
49
=0
=
x 3 + 11x 2 + 31x + 21
lim f ( x )
x → +`
240
8
=
90
3
= lim
3
=
e)
x → −3
x→0
x 3 + 11x 2 + 31x + 21
x → −3
1
x +2
d) lim f ( x )
x → −7
c) lim
x −1
, determina:
c)
x →3
b) lim
x −2
x →2 3
( x − 9 )( x + 3 )
= lim ( x + 3 ) = 6
x →9
x −9
x 3 + 11x 2 + 31x + 21
a) lim
6
= lim
2 −2
x −2
=−
2
x−4
x→4
b)
x −2
= lim
x2 − 3 x + 2
1
6
d) lim
x →2
079
x
7
lim f ( x ) = + `
−
21
→ lim f ( x ) = +
= ` → x →0
`
lim f ( x ) = + ` x → 0
0
+
x →0
=1
=1
421
833276 _ 0392-0451.indd 421
21/7/09 15:43:37
límites y continuidad
080
Si f ( x ) =
x3 + 6 x2 + 9 x
x 2 + 10 x + 21
a) lim f ( x )
b)
lim f ( x )
f)
x→0
x3 + 6 x2 + 9 x
x 2 + 10 x + 21
x →3
e)
x → −3
d) lim f ( x )
x → −7
a) lim
lim f ( x )
c)
x →3
0
, calcula:
=
x3 + 6 x2 + 9 x
b) lim
x → −7
x ( x + 3)
28
x ( x + 3 )2
= lim
=
=`
x
→
−
7
x +7
0
( x + 3 )( x + 7 )
→
x3 + 6 x2 + 9 x
c) lim
d) lim
x →0
e)
081
x3 + 6 x2 + 9 x
x 2 + 10 x + 21
x → −3
x → +`
x → −`
x ( x + 3)
=0
x +7
0
= +`
x 2 + 10 x + 21
x3 + 6 x2 + 9 x
lim
lim f ( x ) = −`
→ No existe lim f ( x ).
x →−7
lim f ( x ) = + `
+
x → −7
x → −7 −
=0
x3 + 6 x2 + 9 x
lim
f)
= lim
x 2 + 10 x + 21
x → −3
lim f ( x )
x → −`
108
9
=
60
5
= lim
x 2 + 10 x + 21
x → −7
lim f ( x )
x → +`
= −`
x 2 + 10 x + 21
Se considera la función f ( x ) =
x
( x −1)2
0
. calcula lim f ( x ) y lim f ( x ).
x →1
x → +`
(Baleares. Junio 2008. Opción A. Cuestión 3)
x
lim
= + `
x →1− ( x − 1)2
1
lim
= =`→
→ lim f ( x ) = + `
x →1 ( x − 1)2
x →1
x
0
lim
= + `
2
+
x →1 ( x − 1)
x
x
lim
x → +`
082
calcula lim
( x − 1)2
=0
x3 − 8 x2 + 7 x
x2 − x
x→0
0
.
(Castilla-La Mancha. Junio 2008. Bloque 1. Pregunta A)
lim
x →0
x3 − 8 x2 + 7 x
2
x −x
= lim
x →0
x ( x − 1)( x − 7 )
= lim ( x − 7 ) = −7
x →0
x ( x − 1)
422
833276 _ 0392-0451.indd 422
21/7/09 15:43:43
Solucionario
083
Se considera la función f ( x ) =
x
x2 −1
7
. calcula lim f ( x ), lim f ( x ) y lim f ( x ).
x → −1
x →1
x → +`
(Baleares. Junio 2008. Opción B. Cuestión 3)
x
lim
= −`
1
x → −1− x 2 − 1
lim
= =`→
→ No existe lim f ( x ).
x → −1 x 2 − 1
x → −1
x
0
lim
= + `
2
+
x → −1 x − 1
x
x
lim
= −`
2
−
1
x →1 x − 1
lim
= =`→
→ No existe lim f ( x ).
2
x →1 x − 1
x →1
x
0
lim
= + `
2
+
x →1 x − 1
x
lim
=0
x → +` x 2 − 1
x
x ).
084
Sea f ( x ) =
2
12
. calcular el límite de la función cuando x tiende a −3.
−
2
x −3
x −9
(Asturias. Septiembre 2002. Bloque 3)
2
12
−
lim
x → −3
x −3
x2 − 9
= lim
x → −3
2 x 2 − 12 x + 18
2 ( x − 3 )2
= lim
= lim
=
x → −3 ( x − 3 )( x 2 − 9 )
x → −3 ( x − 3 )2 ( x + 3 )
lim f ( x ) = −`
−
2
2
→ No existe lim f ( x ).
= = ` → x → −3
x → −3
lim f ( x ) = + `
x+3
0
+
x → −3
1
085
calcula lim ( 2 x + 1) x .
x→0
(La Rioja. Septiembre 2005. Propuesta A. Ejercicio 5)
1
1
lim ( 2 x +1−1) ⋅
x
1
lim ( 2 x + 1) x → 1`
lim ( 2 x + 1) x = e x →0
x →0
x →0
lim
= e x→0
2x
x
= e2
1
086
calcula lim ( cos x ) sen2 x .
x→0
(Navarra. Junio 2001. Opción D. Pregunta 1)
1
2
lim ( cos x ) sen
x
x →0
→ 1`
1
lim ( cos
x →0
2
x ) sen x
=e
lim ( cos x − 1) ⋅
x →0
lim
1
sen2 x
cos x − 1
=e
lim
cos x − 1
x →0
sen2 x
lim
=e
lim
cos x − 1
x → 0 1− cos 2 x
−1
= e x →0 ( 1− cos x ) ( 1 + cos x ) = e x →0 1+ cos x =
1
−
e 2
=
=
1
e
=
e
e
423
833276 _ 0392-0451.indd 423
21/7/09 15:43:48
límites y continuidad
087
x2 − 2
calcula el límite de esta función si x → +`: f ( x ) =
1 + x 2
x2 − 2
lim
x → +`
1+ x 2
x +2
→ 1`
x2 − 2
lim
x → +`
1+ x 2
lim
x +2
2
= e x → +` 1+ x
x 2 −2
=e
088
lim
−1 ⋅ ( x +2 )
=e
lim
x +2
( x 2 −2−1− x 2 ) ( x +2 )
1+ x 2
x → +`
=
−3 x −6
1+ x 2
x → +`
= e0 = 1
4+ x − 4−x
.
4x
calcula lim
x→0
0
(Madrid. Junio 2003. Opción A. Ejercicio 1)
4+ x − 4−x
4 + x −(4 − x)
= lim
x →0 4 x ( 4 + x + 4 − x
4x
lim
x →0
2x
= lim
4 x( 4 + x +
x →0
089
1− 1− x 2
calcula lim
x2
x→0
4−x
)
= lim
x →0
)
=
1
2( 4 + x +
4−x
)
=
1
8
.
(Andalucía. Año 2001. Modelo 4. Opción A. Ejercicio 2)
1− 1− x 2
lim
x →0
x
2
= lim
x →0
= lim
x →0
090
Sabiendo que lim
x→0
a) lim
x 2 ( 1 + 1− x 2
1
1 + 1− x
c) lim
x
x→0
1− cos 2 x
x
x→0
a) lim
x →0
b) lim
2
x →0
d) lim
2
x→0
tg x
x
= lim
x →0
1− cos 2 x
x2
=
)
= lim
x →0
x2
x 2 ( 1 + 1− x 2
)
=
1
2
x
= 1, halla:
sen x
tg x
x→0
b) lim
1− ( 1 − x 2 )
1− cos x
x2
1− cos x
x
sen x
1
= lim
⋅
x → 0
o
sx
x cos x
x
c
sen x
= lim
x →0
sen2 x
x2
0
= 1
sen x sen x
= 1
= lim
⋅
x → 0
x
x
424
833276 _ 0392-0451.indd 424
21/7/09 15:43:53
=
Solucionario
c) lim
1− cos x
x →0
x
2
( 1− cos x )( 1 + cos x )
= lim
2
x ( 1 + cos x )
x →0
= lim
x →0
1− cos 2 x
2
x ( 1 + cos x )
sen2 x
1
= lim
⋅
x → 0
1 + cos x
x2
x ( 1 + cos x )
sen2 x
= lim
x →0
2
7
=
= 1
2
1− cos 2 x
1− cos x
( 1− cos x )( 1 + cos x )
= lim
=
= lim
x →0
x →0
x → 0 x ( 1 + cos x )
x
x ( 1 + cos x )
d) lim
= lim
x →0
091
= 0
x 2 − 1 si x < 3
, determina los límites:
Si g ( x ) = 3
si x ≥ 3
x + 5
a) lim g ( x )
c) lim g ( x )
e)
lim g ( x )
d) lim g ( x )
f)
x → −1
1
8
sen x
sen2 x
sen x
= lim
⋅
x → 0
1 + cos x
x ( 1 + cos x )
x
b)
x →3
x → −5
x→6
lim g ( x )
x → +`
lim g ( x )
x → −`
a) lim g ( x ) = lim ( x 2 − 1) = 0
x → −1
x → −1
b) lim g ( x ) = lim ( x 2 − 1) = 24
x → −5
x → −1
lim g ( x ) = lim ( x 2 − 1) = 8
x → 3−
c)
g ( x ) ≠ lim g ( x ) → No existe lim g ( x ).
3
3 → x lim
x →3
→ 3−
x → 3+
lim g ( x ) = lim
=
x → 3+
x → 3+ x + 5
8
x → 3−
d) lim g ( x ) = lim
x →6
e)
f)
092
x →6
3
3
=
x+5
11
lim g ( x ) = lim
x → +`
x → +`
3
=0
x+5
lim g ( x ) = lim ( x 2 − 1) = + `
x → −`
x → −`
4
si x < −2
x − 2
. calcula estos límites.
Sea la función: h ( x ) = 2
x + 4 x + 4 si −2 ≤ x < 3
x +1
+9
si x > 3
2
a)
lim h ( x )
x → −5
b) lim h ( x )
x →2
c) lim h ( x )
e) lim h ( x )
d) lim h ( x )
f)
x →5
x → −2
x →3
lim h ( x )
x → +`
425
833276 _ 0392-0451.indd 425
21/7/09 15:44:00
límites y continuidad
a) lim h ( x ) = lim
x → −5
x → −5
4
4
=−
7
x −2
b) lim h ( x ) = lim ( x 2 + 4 x + 4 ) = 16
x →2
x →2
c) lim h ( x ) = lim ( 2 x +1 + 9 ) = 73
x →5
x →5
4
= −1
→ lim h ( x ) ≠ lim h ( x )
2
x
−
x → −2
d) x →−2
−
x → −2+
2
lim h ( x ) = lim ( x + 4 x + 4 ) = 0 x → −2
+
+
x → −2
x → −2
→ No existe lim h ( x ).
lim h ( x ) = lim
−
−
x →−2
lim h ( x ) = lim ( x + 4 x + 4 ) = 25
x → 3−
x → 3−
e)
→ lim− h ( x ) = lim+ h ( x )
x → 3
x →3
lim h ( x ) = lim ( 2 x +1 + 9 ) = 25
+
+
x →3
x →3
→ lim h ( x ) = 25
2
x →3
f)
093
lim h ( x ) = lim ( 2
x → +`
x +1
x → +`
+ 9 ) = +`
Dibuja la gráfica aproximada de una función que cumpla simultáneamente
las siguientes condiciones:
lim f ( x ) = 2
lim f ( x ) = 0
x →3
x → −1
lim f ( x ) = −`
lim f ( x ) = 0
x → +`
x → −`
Respuesta abierta. Por ejemplo:
Y
1
1
094
X
Decide si las siguientes funciones son continuas en los puntos que se indican.
En caso de no serlo, determina el tipo de discontinuidad existente.
a) En x = 0 y x = 2.
b) En x = 0 y x = 2.
Y
Y
1
f (x)
1
f (x)
1
X
1
X
426
833276 _ 0392-0451.indd 426
21/7/09 15:44:04
Solucionario
c) En x = 1.
7
e) En x = −2 y x = 2.
Y
Y
f (x)
f (x)
1
1
1
X
X
−1
d) En x = −1 y x = 2.
f ) En x = 1.
Y
Y
f (x)
f (x)
1
1
1
X
X
1
a) • f ( 0 ) = 0 = lim f ( x ) → La función es continua en x = 0.
x →0
n x = 2.
• f ( 2 ) = 4 = lim f ( x ) → La función es continua en
x →2
b) • No existe f ( 0 ).
lim f ( x ) = lim f ( x ) = 0 , 5 → Existe lim f ( x ) = 0 , 5.
x → 0−
x → 0+
x →0
La función no es continua en x = 0, tiene una discontinuidad evitable.
• f ( 2 ) = 2 , 5 = lim f ( x ) → La función es continua en x = 2.
x →2
c) No existe f ( 1 ).
lim f ( x ) = −1
→ lim f ( x ) ≠ lim f ( x ) → No existe lim f ( x ).
x →1
x →1−
x →1+
lim f ( x ) = 3
x →1+
x →1−
La función no es continua en x = 1, tiene una discontinuidad de salto finito.
d) • f ( −1) = 1= lim f ( x ) → La función es continua en
n x = −1.
• f ( 2 ) = 1, 5
x → −1
lim f ( x ) = lim f ( x ) = 2 , 5 → Existe lim f ( x ) = 2 , 5.
x → 2−
x → 2+
x →2
f ( 2 ) ≠ lim f ( x ) → La función no es continua en x = 2, tiene una
x →2
discontinuidad evitable.
e) • No existe f ( −2 ).
lim f ( x ) = + `
→ No
o existe lim f ( x ).
x →−2
lim f ( x ) = −`
x → −2+
x → −2−
La función no es continua en x = −2, tiene una discontinuidad de salto infinito.
427
833276 _ 0392-0451.indd 427
21/7/09 15:44:09
límites y continuidad
• f (2) = 3
lim f ( x ) = −1
→ lim− f ( x ) ≠ lim+ f ( x ) → No existe lim f ( x ).
x →2
x →2
x →2
lim f ( x ) = 3
x → 2+
x → 2−
La función no es continua en x = 2, tiene una discontinuidad de salto finito.
f ) f ( 1) = −1
lim f ( x ) = 2
→ lim− f ( x ) ≠ lim+ f ( x ) → No existe lim f ( x ).
x →1
x →1
x →1
lim f ( x ) = 5
x →1+
x →1−
0
La función no es continua en x = 1, tiene una discontinuidad de salto finito.
095
Estudia la continuidad de las siguientes funciones.
a) y = x 2 − 5 x + 6
b) y =
1
x2 − 5 x + 6
x2 − 4
c) y =
d) y =
4 − x2
e) y = ln x
f ) y = log ( 2 − x )
a) La función es polinómica, por tanto es continua en R.
x = 2
b) x 2 − 5 x + 6 = 0 →
x = 3
Dominio = R − {2, 3}
• No existe f ( 2 ).
lim f ( x ) = + `
→ No exxiste lim f ( x ).
x →2
lim f ( x ) = −`
+
x →2
x → 2−
• No existe f ( 3 ).
lim f ( x ) = −`
→ No exxiste lim f ( x ).
x →3
lim f ( x ) = + `
x → 3+
x → 3−
La función es continua en R −{2, 3}, tiene discontinuidades de salto infinito
en x = 2 y en x = 3.
x ≥ 2
c) x 2 − 4 ≥ 0 → ( x + 2 )( x − 2 ) ≥ 0 →
x ≤ −2
La función está definida en (− `, −2] ∪ [2, + `), por tanto, es continua
en (− `, −2) ∪ (2, + `).
d) 4 − x 2 ≥ 0 → ( 2 + x )( 2 − x ) ≥ 0 → −2 ≤ x ≤ 2
La función está definida en [−2, 2], por tanto, es continua en (−2, 2).
428
833276 _ 0392-0451.indd 428
21/7/09 15:44:13
Solucionario
7
e) No existe f ( 0 ).
lim f ( x ) = −`
→ lim f ( x ) = −
`
lim f ( x ) = −` x →0
x → 0+
x → 0−
La función es continua en R − {0}, tiene una discontinuidad de salto infinito
en x = 0.
f) 2 − x > 0 → x < 2
La función está definida en (− `, 2), por tanto es continua en (− `, 2).
096
¿En qué puntos presentan una discontinuidad estas funciones y de qué tipo son?
a) y =
b) y =
c) y =
5
x −2
6x
x2 − 2 x + 3
3x −6
2
x −2x +1
d) y =
e) y =
f) y =
2x +2
x2 − 2 x − 3
x2 − x
2 x2 + 4 x − 6
2 x2 + 4 x + 6
x2 − x
a) No existe f ( 2 ).
lim f ( x ) = −`
→ No exxiste lim f ( x )
x →2
lim f ( x ) = + `
+
x →2
x → 2−
La función tiene en x = 2 una discontinuidad de salto infinito.
b) x 2 − 2 x + 3 ≠ 0 para cualquier valor de x, así no hay puntos de discontinuidad.
c) x 2 − 2 x + 1 = 0 → x = 1
lim f ( x ) = −`
→ lim f ( x ) = −
`
x →1
lim f ( x ) = −`
x →1+
x →1−
La función tiene en x = 1 una discontinuidad de salto infinito.
x = −1
d) x 2 − 2 x − 3 = 0 →
x = 3
• No existe f ( −1 ).
2 ( x + 1)
2
1
lim f ( x ) = lim
= lim
=−
x → −1
x → −1 ( x + 1)( x − 3 )
x → −1 x − 3
2
• No existe f ( 3 ).
lim f ( x ) = −`
x → 3−
→ No exxiste lim f ( x )
x →3
lim f ( x ) = + `
+
x →3
La función es continua en R − {−1, 3}, tiene una discontinuidad evitable
en x = −1 y una discontinuidad de salto infinito en x = 3.
429
833276 _ 0392-0451.indd 429
21/7/09 15:44:18
límites y continuidad
x = −3
e) 2 x 2 + 4 x − 6 = 0 →
x = 1
• No existe f ( −3 ).
lim f ( x ) = + `
x → −3−
→ No
o existe lim f ( x ).
x →−3
lim f ( x ) = −`
+
x → −3
0
• No existe f ( 1 ).
lim f ( x ) = lim
x →1
x →1
1
x ( x − 1)
x
=
= lim
x →1 2 ( x + 3 )
8
2 ( x − 1)( x + 3 )
La función es continua en R − {−3, 1}, tiene una discontinuidad evitable
en x = −3 y una discontinuidad evitable en x = 1.
x = 0
f ) x 2 − x = 0 →
x = 1
• No existe f ( 0 ).
lim f ( x ) = + `
x → 0−
→ No exxiste lim f ( x ).
x →0
lim f ( x ) = −`
+
x →0
1
• No existe f ( 1 ).
lim f ( x ) = −`
→ No exxiste lim f ( x ).
x →1
lim f ( x ) = + `
+
x →1
x →1−
La función es continua en R − {0, 1}, tiene discontinuidades de salto infinito
en x = 0 y en x = 1.
097
Sea f ( x ) =
2
12
. comprobar si la función es continua en x = 3.
−
2
x −3
x −9
(Asturias. Septiembre 2002. Bloque 3)
No existe f ( 3 ).
2
12
lim
−
2
x → 3
x −3
x −9
2 x 2 − 12 x + 18
2 ( x − 3 )2
= lim
= lim
=
x → 3 ( x − 3 )2 ( x + 3 )
x → 3 ( x − 3 )( x 2 − 9 )
= lim
x →3
1
2
1
=
x+3
3
La función no es continua en x = 3, tiene una discontinuidad evitable.
098
e 3 x − e−3 x
, indica de forma razonada en qué valor x = a no está
4x
definida f ( x ).
Si f ( x ) =
(Castilla-La Mancha. Junio 2007. Bloque 1. Pregunta A)
La función no está definida para los valores que anulan el denominador,
es decir, para x = 0.
430
833276 _ 0392-0451.indd 430
21/7/09 15:44:21
Solucionario
099
7
x + 1 −1
no está definida para x = 0. Definir f (0) de modo
x
que f ( x ) sea una función continua en ese punto.
la función f ( x ) =
(Aragón. Septiembre 2006. Opción B. Cuestión 2)
lim
x →0
x + 1 −1
x + 1− 1
= lim
= lim
x
→
0
x →0 x (
x
x ( x + 1 + 1)
= lim
x →0
1
x +1 +1
=
x
x + 1 + 1)
=
1
2
x + 1 − 1
si x ≠ 0
x
La función es continua si: f ( x ) =
1
si x = 0
2
100
x2 −1
y clasificar sus diferentes
Estudiar la continuidad de la función f ( x ) =
2
x + 3x + 2
tipos de discontinuidad.
(Murcia. Septiembre 2006. Bloque 3. Cuestión A)
x = −2
x 2 + 3 x + 2 = 0 →
x = −1
• No existe f ( −2 ).
lim f ( x ) = + `
x → −2−
→ No
o existe lim f ( x ).
x →−2
lim f ( x ) = −`
x → −2+
• No existe f ( −1 ).
lim f ( x ) = lim
x → −1
x → −1
( x + 1)( x − 1)
x −1
= lim
= −2
x →−1 x + 2
( x + 1)( x + 2 )
La función es continua en R − {−2, −1}, tiene una discontinuidad de salto infinito
en x = −2 y una discontinuidad evitable en x = −1.
101
( 2 x − 1)( x + 2 )
.
¿cuáles son las diferencias entre las funciones y = 2x − 1 e y =
x +2
¿Son las dos funciones continuas?
Si tienen alguna discontinuidad, decide de qué tipo es.
Escribe, si es posible, la segunda función como función definida a trozos utilizando
la primera.
Las funciones tienen la misma gráfica salvo en el punto x = −2. La primera
es una recta y es continua, la segunda está formada por dos semirrectas
y no es continua en este punto.
( 2 x − 1)( x + 2 )
= lim ( 2 x − 1) = −5
x → −2
x+2
La discontinuidad de la segunda función en x = − 2 es evitable.
Así, la segunda función es: f ( x ) = 2 x − 1 si x ≠ −2
lim
x → −2
431
833276 _ 0392-0451.indd 431
21/7/09 15:44:24
límites y continuidad
102
Estudia la continuidad en x = −1 y x = 2 de la función:
si x < −1
3 x − 2
2
f ( x ) = x + 4 x − 1 si −1 ≤ x ≤ 2
11 + ln ( x − 1) si x > 2
clasifica los tipos de discontinuidades.
• f ( −1) = −4
lim f ( x ) = −5
→ No existe lim f ( x ).
x → −1
lim f ( x ) = −4
+
x → −1
x → −1−
La función no es continua en x = −1, tiene una discontinuidad de salto finito.
1
• f ( 2 ) = 11
lim f ( x ) = 11
→ lim f ( x ) = 11 = f ( 2 )
lim f ( x ) = 11 x → 2
+
x →2
x → 2−
La función es continua en x = 2.
103
Estudia la continuidad de la siguiente función en los puntos x = 0 y x = 3.
4
x − 4
g ( x ) = x − 1
1
x −3
si x < 0
si 0 < x ≤ 3
si x > 3
1
• No existe g ( 0 ).
lim g ( x ) = −1
→ lim g ( x ) = −1
x →0
lim g ( x ) = −1
+
x →0
x → 0−
La función no es continua en x = 0, tiene una discontinuidad evitable.
• g( 3 ) = 2
→ No existe lim g ( x ).
x →3
lim g ( x ) = + `
x → 3+
La función no es continua en x = 3, tiene una discontinuidad de salto infinito.
lim g ( x ) = 2
x → 3−
104
Estudia si la función:
x
si x ≤ −1
f ( x ) = 1− x 2 si −1 < x ≤ 2
−3
si 2 < x
es continua en los puntos x = −1 y x = 2.
1
(Castilla-La Mancha. Junio 2005. Bloque 1. Pregunta A)
432
833276 _ 0392-0451.indd 432
21/7/09 15:44:27
Solucionario
7
• f ( −1) = −1
lim f ( x ) = −1
→ No existe lim f ( x ).
x →−1
lim f ( x ) = 0
+
x → −1
x → −1−
La función no es continua en x = −1, tiene una discontinuidad de salto finito.
• f ( 2 ) = −3
lim f ( x ) = −3
→ lim f ( x ) = −3 = f ( 2 )
lim f ( x ) = −3 x → 2
+
x →2
x → 2−
La función es continua en x = 2.
105
Estudiar la continuidad de la función:
x 2 − 9
f ( x ) = x − 3
6
si x ≠ 3
si x = 3
en el punto x = 3.
(Galicia. Junio 2000. Bloque 1. Pregunta 2)
f ( 3) = 6
lim f ( x ) = lim
x →3
x →3
( x − 3 )( x + 3 )
= lim ( x + 3 ) = 6 = f ( 3 )
x →3
x −3
La función es continua en x = 3.
106
Dada la función:
2 x + 5 si x ≤ 1
f ( x ) = 2
x + k si x > 1
determina k para que f ( x ) sea continua en x = 1.
(Castilla-La Mancha. Junio 2001. Bloque 3. Pregunta A)
La función es continua si: lim f ( x ) = f ( 1)
x →1
f ( 1) = 7
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →1
x →1−
x →1+
→ 7 = 1+ k → k = 6
lim f ( x ) = 1 + k
+
x →1
lim f ( x ) = 7
x →1−
107
¿Qué valor debe tomar a en la siguiente función para que sea continua
en el punto x = 4?
cos ( x − 4 ) si x < 4
h ( x ) = x −2 a
2
si x ≥ 4
433
833276 _ 0392-0451.indd 433
21/7/09 15:44:30
límites y continuidad
La función es continua si: lim h ( x ) = h ( 4 )
1
x →4
4−2 a
h( 4 ) = 2
Existe lim h ( x ) si lim h ( x ) = lim h ( x ).
x→4
x→4−
x →4+
→ 24−2a = 1 → 4 − 2 a = 0 → a = 2
lim h ( x ) = 24−2a
+
x→4
lim h ( x ) = 1
x → 4−
108
completa la función para que sea continua en x = 2.
x 2 − 2 x − 1 si x < 2
si x = 2
p ( x ) =
1
si x > 2
x − 3
La función es continua si: lim p ( x ) = p ( 2 )
x →2
Existe lim p ( x ) si lim p ( x ) = lim p ( x ).
x →2
x →2−
x → 2+
lim p ( x ) = −1
→ lim p ( x ) = −1
x →2
lim p ( x ) = −1
+
x →2
x → 2−
2
x − 2 x − 1 si x < 2
si x = 2
Entonces la función es continua si: p ( x ) = −1
1
si x > 2
x − 3
109
1
Sea la función f : R → R dada por:
x 2 − 4 x + 3 si x ≤ 3
f ( x ) =
2 x − 4
si x > 3
¿En qué puntos es continua la función?
(Castilla-La Mancha. Septiembre 2003. Bloque 2. Pregunta A)
La función está formada por dos funciones polinómicas,
por tanto, continuas en los intervalos en los que están definidas.
Estudiamos qué ocurre en el punto x = 3:
f ( 3) = 0
lim f ( x ) = 0
→ No existe lim f ( x ).
x →3
lim f ( x ) = 2
+
x →3
x → 3−
Luego la función no es continua en x = 3, tiene una discontinuidad
de salto finito.
434
833276 _ 0392-0451.indd 434
21/7/09 15:44:34
Solucionario
110
7
Estudia la continuidad de esta función, y especifica los tipos de discontinuidades
que presente.
1 + x 2 si x < −1
si x = −1
f ( x ) = 2
8
si x > −1
3 − x
• f ( x ) = 1 + x 2 es una función polinómica, por tanto, f ( x ) es continua
en (− `, −1).
• f ( −1) = 2
lim f ( x ) = 2
→ lim f ( x ) = 2
x → −1
lim f ( x ) = 2
x → −1+
x → −1−
lim f ( x ) = f ( −1) → f ( x ) es continua en x = 2.
x → −1
8
está definida en R − {3}, por tanto, f ( x ) es continua
3− x
en (−1, 3) ∪ (3, + `).
• f(x)=
• No existe f ( 3 ).
lim f ( x ) = + `
→ No existe lim f ( x ).
x →3
lim f ( x ) = −`
x → 3+
x → 3−
Luego la función no es continua en x = 3, tiene una discontinuidad
de salto infinito.
111
Estudia la continuidad de la función:
3 ln ( x + 2 ) si x < −1
g( x ) = 2
si x = −1
x 2 − 1
si x > −1
• g ( x ) = 3 ln( x + 2 ) está definida en (−2, + `), por tanto, g ( x ) es continua
en (−2, −1).
• g ( −1) = 2
lim g ( x ) = 0
→ lim g ( x ) = 0
lim g ( x ) = 0 x → −1
x → −1+
x → −1−
lim g ( x ) ≠ g ( −1) → g ( x ) no es continua en x = −1, tiene una discontinuidad
x → −1
evitable.
• g ( x ) = x 2 − 1 es una función polinómica, por tanto, g ( x ) es continua
en (−1, + `).
435
833276 _ 0392-0451.indd 435
21/7/09 15:44:38
límites y continuidad
112
Estudia la continuidad de esta función:
4
si x < −2
x + 3
h ( x ) = x 2 + 2 x + 4 si −2 ≤ x < 1
3
si x > 1
x + 7
4
está definida en R − {−3}, por tanto, h ( x ) es continua
x+3
en (− `, −3) ∪ (−3, −2).
• h( x ) =
No existe h ( −3 ).
lim h ( x ) = −`
→ No existe lim h ( x ).
x → −3
lim h ( x ) = + `
+
x → −3
1
x → −3−
Luego la función no es continua en x = −3, tiene una discontinuidad de salto
infinito.
• Estudiamos qué ocurre en el punto x = −2:
h ( −2 ) = 4
lim h ( x ) = 4
→ lim h ( x ) = 4
x → −2
lim h ( x ) = 4
+
x → −2
x → −2−
lim h ( x ) = h ( −2 ) → h ( x ) es continua en x = −2 .
x → −2
• h ( x ) = x 2 + 2 x + 4 es una función polinómica, por tanto, h ( x ) es continua
en (−2, 1).
• h( x ) =
3
está definida en R − {−7} , por tanto, h ( x ) es continua en (1, + `).
x +7
• Estudiamos qué ocurre en el punto x = 1:
No existe h ( 1 ).
lim h ( x ) = 7
x →1−
h ( x ).
3 → No exiiste xlim
→1
lim h ( x ) =
+
x →1
8
Luego la función no es continua en x = 1, tiene una discontinuidad
de salto finito.
113
Estudiar la continuidad de la función:
1
si x ≤ 1
f ( x ) = 2 − x
2
− x + 4 x − 2 si x > 1
(Castilla-La Mancha. Septiembre 2000. Bloque 3. Pregunta A)
436
833276 _ 0392-0451.indd 436
21/7/09 15:44:41
1
`).
Solucionario
7
1
está definida en R − {2}, por tanto, f ( x ) es continua en (− `, 1).
2− x
• f(x)=
• f ( x ) = − x 2 + 4 x − 2 es una función polinómica, por tanto, f ( x ) es continua
en (1, + `).
• Estudiamos qué ocurre en el punto x = 1:
f ( 1) = 1
lim f ( x ) = 1
→ lim f ( x ) = 1
x →1
lim f ( x ) = 1
+
x →1
x →1−
lim f ( x ) = f ( 1) → f ( x ) es continua en x = 1.
x →1
114
Se considera la función real de variable real definida por:
3 x − 2
si x ≥ 2
f ( x ) =
. Estudiar su continuidad.
x ( x − 2 ) si x < 2
(Madrid. Septiembre 2002. Opción A. Ejercicio 2)
• f(x)=
3
x − 2 está definida en R, por tanto, f ( x ) es continua en (2, + `).
• f ( x ) = x ( x − 2 ) es una función polinómica, por tanto, f ( x ) es continua en (− `, 2).
• Estudiamos qué ocurre en el punto x = 2:
f (2) = 0
lim f ( x ) = 0
→ lim f ( x ) = 0
x →2
lim f ( x ) = 0
x → 2+
x → 2−
lim f ( x ) = f ( 2 ) → f ( x ) es continua en x = 2 .
x →2
115
Expresa estas funciones como funciones definidas a trozos, y estudia su continuidad.
a) y = x
c) y = 3 − 2 x
b) y = x + 5
d) y = x 2 − x − 6
e) y = 6 − x 2
x
si x ≥ 0
a) f ( x ) =
− x si x < 0
• f (0) = 0
lim f ( x ) = 0
→ lim f ( x ) = 0
x →0
lim f ( x ) = 0
+
x →0
x → 0−
lim f ( x ) = f ( 0 ) → f ( x ) es continua en x = 0.
x →0
• La función está formada por funciones polinómicas, por tanto,
f ( x ) es continua en R.
437
833276 _ 0392-0451.indd 437
21/7/09 15:44:47
límites y continuidad
x + 5
si x ≥ −5
b) f ( x ) =
− x − 5 si x < −5
• f ( −5 ) = 0
lim f ( x ) = 0
→ lim f ( x ) = 0
x → −5
lim f ( x ) = 0
x → −5+
x → −5−
lim f ( x ) = f ( −5 ) → f ( x ) es continua en x = −5 .
x → −5
• La función está formada por funciones polinómicas, por tanto,
f ( x ) es continua en R.
3 − 2 x si x ≤ 3
2
c) f ( x ) =
3
2 x − 3 si x >
2
3
• f = 0
2
lim f ( x ) = 0
3−
x→
2
→ lim f ( x ) = 0
3
lim f ( x ) = 0
x→
2
3+
x→
2
3
3
lim f ( x ) = f → f ( x ) es continua en x = .
2
3
2
x→
2
• La función está formada por funciones polinómicas, por tanto,
f ( x ) es continua en R.
x = −2
d) x 2 − x − 6 = 0 →
x = 3
1
x 2 − x − 6
si x ≤ −2
2
f ( x ) = − x + x + 6 si − 2 < x ≤ 3
2
x − x − 6
si x > 3
• f ( −2 ) = 0
lim f ( x ) = 0
→ lim f ( x ) = 0
x → −2
lim f ( x ) = 0
+
x → −2
x → −2−
lim f ( x ) = f ( −2 ) → f ( x ) es continua en x = −2 .
x → −2
438
833276 _ 0392-0451.indd 438
21/7/09 15:44:50
Solucionario
7
• f ( 3) = 0
lim f ( x ) = 0
→ lim f ( x ) = 0
x →3
lim f ( x ) = 0
x →3+
x → 3−
lim f ( x ) = f ( 3 ) → f ( x ) es continua en x = 3.
x →3
• La función está formada por funciones polinómicas, por tanto,
f ( x ) es continua en R.
e) 6 − x 2 = 0 → x = ± 6
x 2 − 6 si x ≤ − 6
f ( x ) = 6 − x 2 si − 6 < x ≤ 6
2
x − 6 si x > 6
• f(− 6 ) = 0
f ( x ) = 0
→
lim f ( x ) = 0
+
x →( − 6 )
lim
x →( − 6
)−
lim f ( x ) = 0
x →− 6
lim f ( x ) = f ( − 6 ) → f ( x ) es continua en x = − 6 .
x →− 6
• f( 6 ) = 0
f ( x ) = 0
→ lim f ( x ) = 0
x→ 6
lim f ( x ) = 0
+
x →( 6 )
lim
x →( 6
)−
lim f ( x ) = f ( 6 ) → f ( x ) es continua en x =
x→ 6
6.
• La función está formada por funciones polinómicas, por tanto,
f ( x ) es continua en R.
116
1
. Estudia su continuidad
Se considera la función f ( x ) = sen 4 x −
2
en el intervalo (0, π).
(Cantabria. Junio 2001. Bloque 1. Opción B)
π
π
4 x =
→x=
6
24
1
1
sen 4 x − = 0 → sen 4 x = →
en el intervalo ( 0 , π)
5π
5π
2
2
→x=
4 x =
6
24
1
− sen 4 x si 0 < x < π
2
6
1
π
5π
si
f ( x ) = sen 4 x −
≤x≤
2
6
6
1 − sen 4 x si 5 π < x < π
2
6
439
833276 _ 0392-0451.indd 439
21/7/09 15:44:54
límites y continuidad
π
• f = 0
6
lim f ( x ) = 0
−
π
6
→ lim f ( x ) = 0
π
lim f ( x ) = 0
x→
+
6
π
x→
6
x→
π
π
lim f ( x ) = f → f ( x ) es continua en x = .
6
π
6
x→
6
5π
• f
6
= 0
1
lim f ( x ) = 0
−
5π
6
→ lim f ( x ) = 0
5π
lim f ( x ) = 0
x→
+
6
5π
x→
6
x→
5 π
5π
→ f ( x ) es continua en x =
lim f ( x ) = f
.
6
5π
6
x→
6
La función es continua en (0, π).
117
6 − x
Encontrar el valor de k para el cual la función f ( x ) = 2
2
es continua.
x + kx
(Aragón. Junio 2008. Bloque 3. Opción B)
si x < 2
si x ≥ 2
• La función está formada por funciones polinómicas, por tanto, es continua
en los intervalos en los que están definidas. Estudiamos el punto
en el que cambia su expresión algebraica.
• La función es continua en x = 2 si: lim f ( x ) = f ( 2 )
x →2
f ( 2 ) = 4 + 2k
1
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →2
x → 2−
x → 2+
→ 2 = 4 + 2 k → 2 k = −2 → k = −1
lim f ( x ) = 4 + 2 k
+
x →2
lim f ( x ) = 2
x → 2−
118
Se sabe que la función f : ( −1, +` ) → R definida por:
x 2 − 4 x + 3 si −1 < x < 0
f ( x ) = x2 + a
si x ≥ 0
x + 1
es continua en (−1, +`). Halla el valor de a.
(Andalucía. Junio 2004. Opción B. Ejercicio 1)
440
833276 _ 0392-0451.indd 440
21/7/09 15:44:58
Solucionario
7
Como las funciones son continuas en los intervalos en los que están
definidas, f ( x ) es continua en (−1, + `) si es continua en x = 0,
es decir, si lim f ( x ) = f ( 0 ).
f (0) = a
x →0
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →0
x → 0−
x → 0+
lim f ( x ) = 3
→a=3
lim f ( x ) = a
+
x →0
x → 0−
119
Dada la función:
5 + 2 sen x
si x ≤ 0
f ( x ) =
− x 2 + ax + b si x > 0
¿Para qué valores de los parámetros a y b es continua la función f ( x )?
(Canarias. Junio 2000. Opción A. Cuestión 1)
Para cualquier valor de los parámetros a y b las funciones son continuas
en los intervalos en los que están definidas.
Estudiamos qué ocurre en el punto x = 0:
f (0) = 5
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →0
x → 0−
x → 0+
lim f ( x ) = 5
→b=5
lim f ( x ) = b
x → 0+
x → 0−
Luego f ( x ) es continua si b = 5, independientemente del valor de a.
120
2 x + 1
si x ≤ −2
Dada la función f ( x ) = ax 2 + bx si −2 < x ≤ 4, determina a y b
si 4 < x
x − 4
de modo que sea continua.
(Castilla-La Mancha. Septiembre 2001. Bloque 2. Pregunta A)
• Como las funciones son continuas en los intervalos en los que están definidas,
f ( x ) es continua si es continua en x = −2 y en x = 4.
• La función es continua en x = − 2 si: lim f ( x ) = f ( −2 ) .
x →−2
f ( −2 ) = −3
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x → −2
x → −2−
x → −2+
→ 4 a − 2 b = −3
lim f ( x ) = 4 a − 2 b
+
x → −2
lim f ( x ) = −3
x → −2−
441
833276 _ 0392-0451.indd 441
21/7/09 15:45:02
límites y continuidad
• La función es continua en x = 4 si: lim f ( x ) = f ( 4 )
x →4
f ( 4 ) = 16 a + 4 b
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x→4
x → 4−
x → 4+
lim f ( x ) = 16 a + 4 b
→ 16 a + 4 b = 0
lim f ( x ) = 0
x → 4+
1
4 a − 2 b = −3
a =−
Entonces:
4
→
16 a + 4 b = 0
b = 1
x → 4−
121
calcular los valores de los parámetros a y b para que la función siguiente resulte
continua en todos los puntos.
ax − b
si x < −1
2
f ( x ) = ax − bx + 3 si −1 ≤ x ≤ 2
−bx 3 + a
si x > 2
(Canarias. Junio 2003. Opción B. Cuestión 1)
Como las funciones son continuas en los intervalos en los que están definidas,
f ( x ) es continua si es continua en x = −1 y en x = 2.
• La función es continua en x = −1 si: lim f ( x ) = f ( −1)
x →−1
f ( −1) = a + b + 3
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x → −1
x → −1−
x → −1+
→ − a − b = a + b + 3 → 2 a + 2 b = −3
lim f ( x ) = a + b + 3
+
x → −1
lim f ( x ) = − a − b
x → −1−
1
• La función es continua en x = 2 si: lim f ( x ) = f ( 2 )
x →2
f ( 2 ) = 4 a − 2b + 3
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →2
x → 2−
x → 2+
lim f ( x ) = 4 a − 2 b + 3
→ 4 a − 2 b + 3 = −8 b + a → 3 a + 6 b = −3
lim f ( x ) = −8 b + a
+
x →2
a = −2
2 a + 2 b = −3
Entonces:
→
1
b =
3 a + 6 b = −3
2
x → 2−
122
Sea f : R → R la función continua definida por:
2 − x
si x < a
f ( x ) = 2
x − 5 x + 7 si x ≥ a
donde a es un número real. Determina a.
(Andalucía. Año 2003. Modelo 3. Opción B. Ejercicio 2)
442
833276 _ 0392-0451.indd 442
21/7/09 15:45:08
Solucionario
7
2 − x
si x < a
• Si a ≤ 2, entonces la función es de la forma: f ( x ) = 2
x − 5 x + 7 si x ≥ a
Al ser funciones continuas en los intervalos en los que están definidas,
f ( x ) es continua si es continua en x = a, es decir, si: lim f ( x ) = f ( a )
x →a
f ( a ) = a2 − 5 a + 7
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →a
x → a−
x → a+
→ 2 − a = a2 − 5 a + 7 → a2 − 4 a + 5 = 0
lim f ( x ) = a2 − 5 a + 7
x → a+
lim f ( x ) = 2 − a
x → a−
→ No tiene solución.
si x ≤ 2
2 − x
• Si a > 2 la expresión de la función es: f ( x ) = −2 + x
si 2 < x < a
2
x − 5 x + 7 si x ≥ a
Como las funciones son continuas en los intervalos en los que están definidas,
f ( x ) es continua si es continua en x = 2 y en x = a.
La función es continua en x = 2 porque lim f ( x ) = f ( 2 ) = 0 .
x →2
Estudiamos la función en x = a:
f ( a ) = a2 − 5 a + 7
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →a
x → a−
x → a+
2
2
→ −2 + a = a − 5 a + 7 → a − 6 a + 9 = 0 → a = 3
2
lim f ( x ) = a − 5 a + 7
x → a+
lim f ( x ) = −2 + a
x → a−
123
Para cualquier valor real de a, se considera la función:
x 2 + 2 x
si −` < x ≤ 0
f ( x ) = sen ax
si 0 < x < π
2
( x − π ) + 1 si π ≤ x < +`
Determinar los valores de a para los cuales f ( x ) es continua en todo R.
(Cantabria. Septiembre 2000. Bloque 1. Opción B)
Para cualquier valor de a las funciones son continuas en los intervalos
en los que están definidas. Por tanto, f ( x ) es continua si lo es en x = 0 y en x = π.
• f (0) = 0
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →0
x → 0−
x → 0+
lim f ( x ) = 0
→ lim f ( x ) = 0 = f ( 0 )
x →0
lim f ( x ) = 0
+
x →0
f ( x ) es continua en x = 0 para cualquier valor de a.
x → 0−
443
833276 _ 0392-0451.indd 443
21/7/09 15:45:12
límites y continuidad
• La función es continua en x = π si: lim f ( x ) = f ( π )
x →π
f (π) = 1
lim f ( x ) = sen aπ
π
1
→ sen aπ = 1 → aπ = + 2kπ → a = + 2k , siendo k ∈ Z
lim f ( x ) = 1
2
2
x → π+
x → π−
124
1
considera la función f : ( −` , 10 ) → R definida por:
a x − 6 si x < 2
f ( x ) =
x − 5 si 2 ≤ x < 10
Determina el valor de a > 0 sabiendo que f es continua.
(Andalucía. Año 2001. Modelo 6. Opción A. Ejercicio 1)
Como f ( x ) está formada por funciones continuas en los intervalos
en los que están definidas, es continua en (− `, 10) si lo es en x = 2,
es decir, si: lim f ( x ) = f ( 2 )
f (2) = 3
1
x →2
Existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →2
x → 2−
x → 2+
lim f ( x ) = a2 − 6
→ a2 − 6 = 3 → a2 = 9 → a = ±3
lim f ( x ) = 3
x → 2+
x → 2−
Como a > 0 la función es continua si a = 3.
125
x3 − x2 − 3
se anula en el intervalo [1, 3].
2x +1
Menciona los resultados teóricos en que te apoyas para hacer tus afirmaciones.
Demuestra que la función f ( x ) =
1
f ( x ) es continua en R − −
2
f ( 1) = −1 < 0
1
, luego es continua en [1, 3].
15
>0
7
Aplicamos el teorema de Bolzano → Existe c ∈ (1, 3), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (1, 3).
f ( 3) =
126
1
Sea f ( x ) = 2 − x + ln x con x ∈ (0, +`). Probar que existe un punto c ∈
, 1
e 2
tal que f ( c ) = 0.
(Castilla y León. Septiembre 2008. Prueba B. Problema 2)
1
f ( x ) es continua en (0, + `), luego es continua en
, 1 .
e 2
1
1
=−
<0
f
e 2
e2
f ( 1) = 1 > 0
444
833276 _ 0392-0451.indd 444
21/7/09 15:45:18
Solucionario
7
1
Aplicamos el teorema de Bolzano → Existe c ∈
, 1 , tal que f ( c ) = 0,
e 2
1
es decir, la función se anula en algún punto del intervalo
, 1 .
e 2
k∈Z
127
Demuestra que existe al menos un número real x para el que se verifica sen x = x − 2.
(Baleares. Septiembre 2001. Opción B. Cuestión 1)
Consideramos la función f ( x ) = sen x − x + 2.
f ( x ) es continua en R, luego es continua en [2, 3].
f ( 2 ) = sen 2 = 0,909 > 0
f ( 3 ) = sen 3 − 1 = − 0,858 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (2, 3), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (2, 3),
por tanto, la ecuación tiene al menos una solución en este intervalo.
128
Determinar si el polinomio x 4 − 4x 2 − 1 tiene alguna raíz real negativa.
(Extremadura. Junio 2003. Repertorio B. Ejercicio 1)
Consideramos la función f ( x ) = x 4 − 4 x 2 − 1.
f ( x ) es continua en R, luego es continua en [−3, −2].
f ( −3 ) = 44 > 0
f ( −2 ) = −1 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (−3, −2), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (−3, −2),
luego el polinomio tiene alguna raíz real negativa.
129
Se considera la ecuación x 3 + x 2 + mx − 6 = 0. utilizando el teorema de Bolzano,
demuestra:
a) Si m > −3 entonces la ecuación tiene al menos una raíz real menor que 2.
b) Si m < −3 entonces la ecuación tiene al menos una raíz real mayor que 2.
(Baleares. Junio 2003. Opción B. Cuestión 3)
a) Consideramos la función f ( x ) = x 3 + x 2 + mx − 6.
f ( x ) es continua en R, luego f ( x ) es continua en [0, 2].
f ( 0 ) = −6 < 0
f ( 2 ) = 2 m + 6 > 0 → 2 m > −6 → m > −3
Aplicamos el teorema de Bolzano → Existe c ∈ (0, 2), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 2),
por tanto, la ecuación tiene al menos una raíz real menor que 2.
b) Consideramos la función f ( x ) = x 3 + x 2 + mx − 6 continua en R.
f ( 2 ) = 2 m + 6 < 0 → 2 m < −6 → m < −3
Al ser lim f ( x ) = + ` → Existe un valor b > 2, tal que f ( x ) es continua
x → +`
en [2, b] y f ( b ) > 0.
Entonces, aplicando el teorema de Bolzano, tenemos que existe c ∈ (2, b),
tal que f ( c ) = 0, es decir, la función se anula en algún punto del intervalo (2, b)
con b > 2, por tanto, la ecuación tiene al menos una raíz real mayor que 2.
445
833276 _ 0392-0451.indd 445
21/7/09 15:45:21
límites y continuidad
130
1
Probar que la ecuación x = cos x tiene solución positiva.
(Extremadura. Septiembre 2004. Repertorio B. Ejercicio 1)
Consideramos la función f ( x ) = x − cos x.
f ( x ) es continua en R, luego f ( x ) es continua en [0, 1].
f ( 0 ) = −1 < 0
f ( 1 ) = 1 − cos 1 = 0,459 > 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0, 1), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 1),
por tanto, la ecuación tiene al menos una solución positiva.
131
¿Puede asegurarse, utilizando el teorema de Bolzano, que la función f ( x ) = tg x
π 3π
? razona la respuesta.
tiene una raíz en el intervalo ,
4 4
(Galicia. Junio 2003. Bloque 3. Pregunta 2)
Consideramos la función f ( x ) = tg x .
π 3π
π
, por tanto, la función no es continua en ,
4 4
2
y no puede aplicarse el teorema de Bolzano, así que no puede asegurarse
que la función tenga una raíz en este intervalo.
f ( x ) no está definida en x =
132
1
calcular, con un error menor que una décima, una raíz positiva
del polinomio x 3 + x − 1.
(Extremadura. Septiembre 2001. Repertorio A. Ejercicio 1)
Consideramos la función f ( x ) = x 3 + x − 1 continua en R.
f (0) = − 1 < 0
f (1) = 1 > 0
Como f ( x ) es continua en [0, 1] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0, 1), tal que f ( c ) = 0.
1
f ( 0,5 ) = −0,375 < 0
Como f ( x ) es continua en [0,5; 1] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0,5; 1), tal que f ( c ) = 0.
f ( 0,9 ) = 0,629 > 0
Como f ( x ) es continua en [0,5; 0,9] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0,5; 0,9), tal que f ( c ) = 0,184.
f ( 0,6 ) = −0,184 < 0
Como f ( x ) es continua en [0,6; 0,9] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0,6; 0,9), tal que f ( c ) = 0.
f ( 0,7 ) = 0,043 > 0
Como f ( x ) es continua en [0,6; 0,7] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0,6; 0,7), tal que f ( c ) = 0.
446
833276 _ 0392-0451.indd 446
21/7/09 15:45:24
Solucionario
133
7
Demuestra que existe un punto x = c en el que la función f ( x ) = x 2 + x ⋅ 2 x toma
el valor 2. Encuéntralo, aproximando su expresión hasta las centésimas.
Si f ( c ) = 2 → f ( c ) − 2 = 0
Consideramos la función g ( x ) = x 2 + x ⋅ 2 x − 2.
g ( x ) es continua en R, luego g ( x ) es continua en [0, 1].
g ( 0 ) = −2 < 0
g (1) = 1 > 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0, 1), tal que g( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 1),
por tanto, f ( c ) − 2 = 0 → f ( c ) = 2.
g ( 0,5 ) = −1,043 < 0
g ( 0,7 ) = −0,372 < 0
g ( 0,9 ) = 0,489 > 0
g ( 0,75 ) = −0,176 < 0
g ( 0,6 ) = −0,73 < 0
g ( 0,79 ) = −0,009 < 0
g ( 0,8 ) = 0,032 > 0
Como g ( x ) es continua en [0,79; 0,8] podemos aplicar el teorema
de Bolzano → Existe c ∈ (0,79; 0,8), tal que g ( c ) = 0, por tanto, f ( x ) toma
el valor 2 en algún punto del intervalo (0,79; 0,8).
134
Dadas las funciones f ( x ) = x sen x y g ( x ) = ln x, justifica que existe un punto
del intervalo [2, 3] donde ambas funciones toman el mismo valor.
Consideramos la función h ( x ) = x sen x − ln x.
f ( x ) es continua en R y g ( x ) es continua en (0, + `), por tanto, h ( x ) es continua
en [2, 3].
h ( 2 ) = 1,125 > 0
h ( 3 ) = −0,675 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (2, 3), tal que h ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (2, 3),
por tanto, h ( c ) = f ( c ) − g ( c ) = 0 → f ( c ) = g ( c ).
135
1
se cortan
Demuéstrese que las gráficas de las funciones f ( x ) = e x y g ( x ) =
x
en un punto x > 0.
(Castilla y León. Junio 2004. Prueba A. Cuestión 2)
1
.
x
f ( x ) es continua en R y g ( x ) es continua en R − {0}, por tanto, h ( x ) es continua
en R − {0}.
Consideramos la función h ( x ) = e x −
h ( 0,5 ) = −0,351 < 0
h ( 1 ) = 1,718 > 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0,5; 1), tal que h ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0,5; 1),
por tanto, h ( c ) = f ( c ) − g( c ) = 0 → f ( c ) = g ( c ), es decir, las funciones
se cortan en un punto de este intervalo.
447
833276 _ 0392-0451.indd 447
21/7/09 15:45:26
límites y continuidad
136
π
Dada la función f ( x ) = x sen x , demuestra que existe α ∈ (0, 4)
4
tal que f (α ) = f (α + 1). Menciona los resultados teóricos que utilices.
(Navarra. Junio 2007. Grupo 2. Opción C)
π
π
Consideramos la función g ( x ) = x sen x − ( x + 1) sen ( x + 1) .
4
4
f ( x ) es continua en R, por tanto, g ( x ) es continua en [0, 4].
2
<0
2
5 2
>0
2
Aplicamos el teorema de Bolzano → Existe α ∈ (0, 4), tal que g ( α ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 4),
por tanto: g ( α ) = f ( α ) − f ( α + 1) = 0 → f ( α ) = f ( α + 1)
g( 0 ) = −
g( 4 ) =
PREPARA TU SELECTIVIDAD
1
calcula:
a)
2+n
lim
n → +`
1+ n
1−5 n
b)
n 4 + 2 n3 − 3 − n 4 − n
n+5
lim
n → +`
(Madrid. Año 2008. Modelo. Opción A. Ejercicio 2)
a)
2+n
lim
n→ +`
1+ n
1− 5 n
→ 1`
2+n
lim
n→ +`
1+ n
−1 ⋅ ( 1− 5 n )
lim
lim
1− 5 n
n→ +
= e ` 1+ n
= e n→ +`
2+ n
lim
1−5 n
= e n→ +` 1+ n = e−5 =
b)
lim
n4 + 2 n3 − 3 − n4 − n
=
n+5
= lim
n→ +`
= lim
n→ +`
= lim
n→ +`
(
n4 + 2 n3 − 3 − n4 − n
)(
n4 + 2 n3 − 3 + n4 − n
( n + 5 )( n + 2 n − 3 + n − n
4
=
1
n4 + 2 n3 − 3 − n4 − n
→`−`
n+5
n→ +`
1+ n
e5
lim
n→ +`
( 2 + n − 1− n )( 1− 5 n )
3
4
n4 + 2 n3 − 3 − n4 + n
( n + 5 )( n4 + 2 n3 − 3 + n4 − n
)
2 n3 + n − 3
( n + 5 )( n4 + 2 n3 − 3 + n4 − n
)
)
)
=
=
=1
448
833276 _ 0392-0451.indd 448
21/7/09 15:45:31
Solucionario
2
7
calcule:
a)
lim
n → +`
(
n2 − 5 n + 4 − n )
b)
2n − 8
lim
2 n+1
n → +`
(Galicia. Septiembre 2005. Bloque 4. Pregunta 1)
a)
lim
(
n2 − 5 n + 4 − n ) → ` − `
lim
(
n2 − 5 n + 4 + n ) = lim
n→ +`
n→ +`
2n − 8
lim
n→ +`
2n+1
n2 − 5 n + 4 + n
n2 − 5 n + 4 − n2
n→ +`
3
n2 − 5 n + 4 + n )( n2 − 5 n + 4 + n )
n→ +`
= lim
b)
(
2
n − 5n + 4 + n
3
n
= lim 2 − 2
n→ +` 2n+1
2n+1
= lim
n→ +`
−5 n + 4
2
n − 5n + 4 + n
2
= lim 1 − 2
n→ +` 2
2n
=
=−
5
2
= 1
2
Determina el valor de a para el cual:
lim
x → +`
(2x −
4 x 2 + ax + 1 ) = 1
(La Rioja. Junio 2000. Propuesta A. Ejercicio 4)
lim
x → +`
( 2 x −x →lim+4`(x22 +x −ax +4 x12)+=ax + 1 ) =
= lim
x → +`
( 2=x −lim 4 (x22 +x −ax +4 x12)+( 2axx ++ 1 4)(x22 x++ax +4 x12)+ ax + 1 ) =
x → +`
= lim
x → +`
=−
4
2x +
4 x 2 − 4 x 2 − ax − 1
2x +
2
4 x + ax + 1
= lim
x → +`
=
− ax − 1
4 x 2 + ax + 1
2x +
=
a
= 1 → a = −4
4
Determina el valor de a para el cual:
x +3
lim
x → +`
x
=
4 x12 + ax + 1
4 x22 x++ax +
ax
= e
(La Rioja. Junio 2001. Propuesta A. Ejercicio 4)
x+3
lim
x → +`
x
ax
→ 1`
x+3
lim
x → +`
x
lim
ax
= e x→ +`
x +3
x
−1 ⋅ ax
lim
= e x → +`
= e3 a = e → 3 a = 1 → a =
( x +3− x ) ⋅ ax
x
lim
= e x → +`
3 ax
x
=
1
3
449
833276 _ 0392-0451.indd 449
21/7/09 15:45:35
límites y continuidad
5
Estudia si la función:
x
si x ≤ −1
f ( x ) = 1− x 2 si −1 < x ≤ 2
si 2 < x
−3
es continua en los puntos x = −1 y x = 2.
(Castilla-La Mancha. Junio 2005. Bloque 1. Pregunta A)
• f ( −1) = −1
lim f ( x ) = −1
→ No exiiste lim f ( x ) → f ( x ) no es continua en x = −1.
x → −1
lim f ( x ) = 0
x → −1+
x → −1−
La función tiene una discontinuidad de salto finito en el punto x = −1.
• f ( 2 ) = −3
lim f ( x ) = −3
→ lim f ( x ) = −3 = f ( 2 ) → f ( x ) es continua en x = 2.
x →2
lim f ( x ) = −3
x → 2+
x →2−
6
Determinar los valores de a y b para que la función siguiente sea continua
en todos los puntos.
ax 2 + b si x < 0
si 0 ≤ x < 1
f ( x ) = x − a
a
+ b si 1 ≤ x
x
(Canarias. Junio 2004. Opción A. Cuestión 1)
f ( x ) está formada por dos funciones polinómicas, por tanto, continuas,
y una función racional que no está definida en x = 0, pero que es continua
en el intervalo (1, + `). Así la función es continua en todos los puntos
si lo es en los puntos en los que cambia su expresión algebraica.
• La función es continua en x = 0 si: lim f ( x ) = f ( 0 )
x →0
f ( 0 ) = − a y existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →0
lim f ( x ) = b
x → 0−
→ b = −a
lim f ( x ) = − a
x → 0+
x → 0−
x → 0+
• La función es continua en x = 1 si: lim f ( x ) = f ( 1)
x →1
f ( 1) = a + b y existe lim f ( x ) si lim f ( x ) = lim f ( x ).
x →1
x →1−
x →1+
lim f ( x ) = 1− a
→ 1− a = a + b
lim f ( x ) = a + b
+
x →1
x →1−
Entonces:
a = 1
b = − a
→
2 a + b = 1
b = −1
450
833276 _ 0392-0451.indd 450
21/7/09 15:45:40
Solucionario
7
7
Busca algún criterio que te permita afirmar que la ecuación:
x3 + x2 − 7 x + 1 = 0
tiene al menos una solución en el intervalo (0, 1). ¿Qué te dice ese criterio
para el intervalo (−1, 0)? razona la respuesta.
(La Rioja. Septiembre 2007. Propuesta A. Ejercicio 3)
Consideramos la función f ( x ) = x 3 + x 2 − 7 x + 1.
f ( x ) es continua en R, luego f ( x ) es continua en [0, 1].
f (0) = 1 > 0
f ( 1 ) = −4 < 0
Aplicamos el teorema de Bolzano → Existe c ∈ (0, 1), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (0, 1),
luego la ecuación tiene alguna solución en este intervalo.
f (−1) = 8 > 0 → No podemos aplicar el teorema de Bolzano en (−1, 0)
porque f ( 0 ) y f (−1) no tienen signos distintos.
8
Demostrar que la ecuación x 3 + x − 5 = 0 tiene al menos una solución
en el intervalo (1, 2).
(Castilla y León. Junio 2008. Prueba B. Cuestión 3)
Consideramos la función f ( x ) = x 3 + x − 5.
f ( x ) es continua en R, luego f ( x ) es continua en [1, 2].
f (1) = − 3 < 0
f (2) = 5 > 0
Aplicamos el teorema de Bolzano → Existe c ∈ (1, 2), tal que f ( c ) = 0,
es decir, la función se anula en algún punto del intervalo (1, 2),
por tanto, la ecuación tiene al menos una solución en este intervalo.
451
833276 _ 0392-0451.indd 451
21/7/09 15:45:41
© Copyright 2025