1. No category

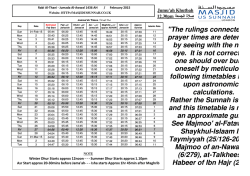
Dear Parents/Guardians,
As every teacher knows, stude ts ho do ’t
ai tai regular a ade i pursuits over the summer
months significantly regress in the knowledge and skills they gained over the previous year. In an effort to
combat this, students are being provided an age-appropriate Math Activity Log to complete over the course
of the summer as well as a Review Assessment. These assignments are to be turned in on the first day of
school. We are asking parents/guardians to oversee an allotted amount of time working with their children on
the identified skills every week. After your students complete their weekly math work, please record the
amount of time spent and the material they covered on the activity log.
See the attached Activity List for grade-appropriate tasks that students can use to fulfill their weekly
minute requirement. Students entering grade 5 and above need to fulfill their Activity Log hours by
completing course work through KhanAcademy.org or TenMarks.com. The websites are free, easy to use
resources that cover a wide range of math content. Please supervise your children while using the internet.
The review assessment is an assignment that helps a student show their mastery of the content
covered this year in math. While these topics were chosen to be review, students are encouraged to use their
previous notes or online resources to help them refresh their knowledge of this content. Just completing this
review is not enough to earn full credit on the summer assignment. Students must be engaged with math all
su
er, as e ide ed
their a ti it log. Co pletio of the re ie
ill help deter i e a stude t’s
le el for their e t ear, so it’s i porta t that e see their u dersta di g of the
ath
aterial.
It is our sincere hope that this format for summer math work is more enjoyable for students, easier for
parents to oversee, and more effective in maintaining students' skills over the summer. With that in mind,
ensuring that your child completes their weekly work is one of the most effective ways to prepare them for
success in the coming school year.
Have a relaxing and productive summer!
Queridos Padres/ Guardianes,
Como cualquier maestro sabe, los estudiantes que no mantienen búsqueda regular académico durante
los meses del verano retroceden significamente en el conocimiento y las habilidades que han ganado en el año
previo. En el esfuerzo de poder combatir eso, les hemos dado a los estudiantes con la edad apropiada un Math
Activity Log donde debe estar completada durante el transcurso del verano y también un Review
Assesment. Estos trabajos deben der ser entregados el primer día de la escuela. Le estamos pidiendo a los
padres/guardines que supervisen el tiempo asignado de trabajo con sus niños en las habilidades
identificadas cada semana. Después que los estudiantes terminen el trabajo semanal, por favor registre el
tiempo en que duraron y el material que hicieron en el registro de actividades.
Mira la forma Activity List para la tarea, con el nivel apropiado en donde los estudiantes puedan
cumplir su requerimiento semanal. Estudiantes entrando al quinto grado y más necesitan que cumplir sus
horas del Activity Log por completar el trabajo del curso en KhanAcademy.org o TenMarks.com. Las paginas so
gratis, y el uso es fácil para usar los recursos que se extiende en el contento de matemáticas. Por favor
supervise sus niños/ niñas cuando usen el Internet.
El review assesment (revisión de valoración) es un asignación en donde ayuda al estudiante mirar su
dominio de los contenidos este ano en matemáticas. Mientras estos temas fueros seleccionados para revisión,
animamos a los estudiantes que usen las notas previas o recursos por Internet para que les ayuden refrescar
su conocimiento de este contenido. No más en completar la revisión, no es lo suficiente en recibir un crédito
completo para su activity log. En completar la revisión nos ayudara determinar el nivel del estudiante para el
próximo ano, por esa razón es muy importante que nosotros miremos el entendimiento del material.
Es nuestra honesta esperanza que este formato para el trabajo de matemáticas durante el verano es
algo más agradable para los estudiantes, fácil para que los padres supervisen y más eficiente en mantener las
habilidades de los estudiantes durante el verano. Con eso en la mente, asegurándose que su niño/niña
termine el trabajo semanal es una de las maneras eficientes en prepararlos al éxito para el próximo año
escolar.
Que tengan un verano relajante y productivo!
Summer 2015 Math
Minimum Skills List
Choose the grade level your student will be entering next school year.
These are the minimum skills to practice; helpful websites are at the end of this document.
For the younger grades, we suggest working directly with your child on the objectives listed; ask
you kindergartner to count from 50 to 100, or practice multiplication facts with your 4th grader.
Students entering 4th grade or above are strongly encouraged to spend their time on the free
Khan Academy website or the new TenMarks.com program; for those without access to the
internet or a library, we suggest practicing multiplication facts or picking something from the
"Other Ideas" section.
Kindergarten










count to 10 (goal is 100)
count objects to 10
recognize that a number can tell how many of something - example: show me 3 toys (from
0-10), how many people are in the room, house, family etc
recognizing numbers from 1-10 by value (name)
recognizing shapes by name (triangle, circle, square, rectangle)
recognizing colors (red, orange, yellow, green, blue, purple, black, white)
putting events in order (first I got out of bed, second I got dressed, third I ate breakfast) or
in the story this happened, then this happened, then this happened
sorting by color, size, weight
recognizing things that are the same or different
follow directions to arrange or position objects (for example: put your pants away in your
bottom drawer)
First Grade











Sorting by color, size, shape etc: Sorting Laundry, Socks, Silverware
Recognizing if something is larger or smaller, heavier or lighter, shorter or taller, bigger or
smaller, left or right
(small, smaller, smallest) (tall, taller, tallest) (big, bigger, biggest) (left, middle, right) (top,
bottom, between)etc.
recognizing simple patterns, creating simple patterns (numbers, shapes, colors, or words)
recognizing triangles, circles, squares, rectangles, spheres, cubes, and other shapes
Telling time to the hour and half hour
Counting up to 100 by 1's, 2's, 5's, 10's
Counting up or down from a number under 100
Addition facts to 10
Subtraction facts from 10
writing numbers from 1-100 having only 10 numbers per line:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
etc
Recognizing coin money and the value of each: pennies, nickels, dimes, and quarters.
- more advanced skill counting money
Second Grade

Mastery of all kindergarten and First grade skills



Counting money (mainly coins)
Know place value up to the thousands place
Reading analog clocks to the quarter hour (fifteen minutes)
Third Grade










ALL Kindergarten-second grade skills
Multiplication tables of 0's, 1's, 2's,3's, 4's, 5's, 10's
Skip counting of 1's, 2's, 3's, 5's, 10's- 4's as well, but 4's can come last.
Mastery of addition and subtraction to 10 (know all combinations of numbers that make 10
Practice addition and subtraction with two digit and three digit numbers
Reading time in five minute intervals (examples-12:05, 3:55) using an analog clock
Units of measurement
Money: know coins that add up to $1.00.
Fractions: recognizing a fraction as part of a hole
Place value to the thousands place
- practice understanding that 10 'ones' is the same as one 'ten'; ten 'tens' is the same as
one 'hundred'; ten 'hundreds' is the same as one 'thousand'
Fourth Grade






ALL Kindergarten-third grade skills
Addition and subtraction facts
Multiplication tables to 144 (up to 12x12)
Division tables from 144
Know divisibility rules for 2, 5, 10
Reading an analog clock to the minute
Fifth Grade





All K-4 skills, especially memorization of multiplication tables
Mastery of multiplication and division facts
Know divisibility rules for 2,3,5,9,10
Fractions with addition, subtraction, multiplication and division
Solving one-step algebra problems
Sixth Grade






All K-5 skills, especially mastery of fractions
Mastery of multiplication and division facts
Multiply and divide by 10, 100, and 1000 etc. using mental math
Operations on decimals and fractions (add, subtract, multiply, divide)
Order of operations
Solving algebra problems with a one variable on one side of the equation.
Seventh Grade





All K-6 skills, especially memorization of multiplication tables
Locating integers (positive and negative), fractions, and decimals on a number line
Recognizing prime numbers
Order of operations with positive numbers, fractions, and decimals
Writing an algebraic equation from a word problem

Solving algebra problems with a variable on both sides of the equation.
Eighth Grade



All K-7 skills, especially operations on integers (positive and negative), fractions, and decimals
Prime factorization
GCF, LCM

Order of operations with integers (positive and negative numbers), fractions, and decimals


Solving equations with variables
Solving word problems using variables
High School


All K-8 skills are necessary
KhanAcademy.org or TenMarks.com are required for all High School students. For
assistance with these websites, email [email protected].
Other Ideas that will count as math time:
Math related Games: Flash Cards, Suduko Puzzles, UNO, Go Fish, Rummy, War, Board Games,
Yatzee, etc.
Chores that involve math skills: sorting socks, putting toys away by sorting, cooking if any type
of measurement is involved, grocery shopping, determining a tip at a restaurant, etc.
Useful Websites:
At the time this was created, the websites listed were checked by teachers and deemed child
appropriate. However, parents should always monitor their child's use of any Internet site.
KhanAcademy.org & TenMarks.com – These websites are highly recommended for students
entering the 4th grade or higher. They are required for high school students.
aaamath.com
aplusmath.com/games/index.html
coloringsquared.com
coolmath4kids.com
funbrain.com
kidsnumbers.com
lizardpoint.com/math/
math.com
math-aids.com
math-drills.com
mathisfun.com
mathforum.org
pbskids.org/games/math/
*Mainly for up to grade 2
purplemath.com
weeklyreader.com/kids/games/sudoku.asp
youcubed.com
Name:___________________
6th - 11th Grade Summer Math Activity Log
Math Activity Logs due August 31, 2015
Please return completed logs to your math class on the first day of school.
Students will record the number of minutes they are active each day and the activity they did.
They are striving to reach the 60 minute weekly requirement.
Parents/Guardians must sign the log at the end of each month.
Registro de actividad de Matemáticas se entrega el 25 de Agosto
Devolver los registros completados el primer día de clases.
Los estudiantes apuntarán cuántos minutos estudiaron cada día y cual actividad hicieron.
Ellos deben estudiar 60 minutos cada semana.
Los padres deben firmar el registro al final de cada mes.
June
Sun, 5/31
Mon, 6/1
Tue, 6/2
Wed, 6/3
Thu, 6/4
Fri, 6/5
Sat, 6/6
Total minutes
Sun, 6/7
Mon, 6/8
Tue, 6/9
Wed, 6/10
Thu, 6/11
Fri, 6/12
Sat, 6/13
Total minutes
Sun, 6/14
Mon, 6/15
Tue, 6/16
Wed, 6/17
Thu, 6/18
Fri, 6/19
Sat, 6/20
Total minutes
Sun, 6/21
Mon, 6/22
Tue, 6/23
Wed, 6/24
Thu, 6/25
Fri, 6/26
Sat, 6/27
Total minutes
Week 1
Week 2
Week 3
Week 4
Students have a goal to practice 240 minutes in June.
(Estudiantes tienen una meta de practicar 240 minutos el mes de Junio.)
Parent/Guardian Signature:___________________________________________
(Padres firman aqui)
July
Sun, 6/28
Week 5
Mon, 6/29
Tue, 6/30
Wed, 7/1
Thu, 7/2
Fri, 7/3
Sat, 7/4
Total minutes
July (continued)
Sun, 7/5
Mon, 7/6
Tue, 7/7
Wed, 7/8
Thu, 7/9
Fri, 7/10
Sat, 7/11
Total minutes
Sun, 7/12
Mon, 7/13
Tue, 7/14
Wed, 7/15
Thu, 7/16
Fri, 7/17
Sat, 7/18
Total minutes
Sun, 7/19
Mon, 7/20
Tue, 7/21
Wed, 7/22
Thu, 7/23
Fri, 7/24
Sat, 7/25
Total minutes
Sun, 7/26
Mon, 7/27
Tue, 7/28
Wed, 7/29
Thu, 7/30
Fri, 7/31
Sat, 8/1
Total minutes
Week 6
Week 7
Week 8
Week 9
Students have a goal to practice 300 minutes in July.
(Estudiantes tienen una meta de practicar 300 minutos el mes de Julio.)
Parent/Guardian Signature:___________________________________________
(Padres firman aqui)
August
Sun, 8/2
Mon, 8/3
Tue, 8/4
Wed, 8/5
Thu, 8/6
Fri, 8/7
Sat, 8/8
Total minutes
Sun, 8/9
Mon, 8/10
Tue, 8/11
Wed, 8/12
Thu, 8/13
Fri, 8/14
Sat, 8/15
Total minutes
Sun, 8/16
Mon, 8/17
Tue, 8/18
Wed, 8/19
Thu, 8/20
Fri, 8/21
Sat, 8/22
Total minutes
Week 10
Week 11
Week 12
Students have a goal to practice 180 minutes in August.
(Estudiantes tienen una meta de practicar 180 minutos el mes de Augusto.)
Parent/Guardian Signature:___________________________________________
(Padres firman aqui)
Name:
Date:
End-of-Course Test A
Multiple Choice
100
(10 3 2 points 5 20 points)
Suggested Time:
90 min
Fill in the circle next to the correct answer.
1. The recorded value of a measurement, to the nearest 1,000, is 870,000.
How many significant digits are in this measurement?
2 significant digits
A
3 significant digits
B
4
significant digits
C
5
significant digits
D


2. Simplify 215 1 1 1 2 1 1  .
8
2 
2
22
C
33
160
3. Factor 218a 1 90b 2 36 completely.
22(9a 1 45b 2 18)
A
9(22a 1 10b 1 4)
C
5
8
B
1
D
24
B
D
6(23a 2 15b 2 6)
218(a 2 5b 1 2)
3
8
4. Marilyn plans to buy a book to read during her summer vacation. She has at
most $18 set aside to purchase the book. The sales tax in her state is 8%. If
x is the maximum price of book that she can buy to stay within her budget,
which of the following inequalities can be used to find the value of x?
x 1 0.08x , 18
x 1 0.08x f 18
A
B
x 1 0.08x . 18
x 1 0.08x v 18
C
D
5. Which one of the following equations represent a direct proportion?
A y 5 3x 1 5
B y 5 12
C
x
3
2y 5 x
5
D xy 5 18.6
150
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
© Marshall Cavendish International (Singapore) Private Limited.
7
8
A
Name:
Date:
of a triangle is always 180°.
6. The sum of the measures of the
interior angles
A
exterior angles
B
corresponding angles
C
alternate angles
D
A cylindrical bottle full of juice has a base radius of 3 inches and a height
of 10 inches. The bottle tipped over and some juice spilled out. Now the bottle is only 2 full.
5
How many cubic inches of juice spilled out? Use 3.14 as an approximation for U.
7.
A
C
84.78 in3
254.34 in3
B
D
169.56 in3
423.9 in3
8. A plan of Vera’s bedroom is drawn using a scale of 1 : 50. If the actual length of
her bedroom window is 1.8 meters, what is the length of the window on the plan?
0.36 cm
0.9 cm
A
B
3.6 cm
9.0 cm
C
D
9. When Q2 , mean , Q3, which of the following statements is true?
More than 50% of the data values are greater than the mean.
A
The difference between the mean and Q1 is less than the
B
interquartile range.
The number of data values that lie between the median and the mean is 25%.
C
The number of data values less than the mean does not exceed 75%.
D
© Marshall Cavendish International (Singapore) Private Limited.
10. The table shows the probability distribution of a town’s population by age group.
Age Group
(in years)
Less than 20
20–39
40–60
Above 60
Probability
17.5%
36%
24.8%
21.7%
You randomly select a person that resides in the town. Which of the following
statements is correct?
A The probability of selecting a person between 35 and 50 years old is not
more than 60.8%.
B The probability of selecting a person between 25 and 35 years old is 36%.
C The probability of selecting a person less than 30 years old is 53.5%.
D The probability of selecting a person of 45 years or older is at least 46.5%.
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
151
Name:
Date:
Short Answer and/or Constructed Response
(Questions 11 to 20: 10 3 2 5 20 points, Questions 21 to 23: 3 3 4 5 12 points,
Questions 24 to 27: 4 3 5 5 20 points, Questions 28 to 31: 4 3 6 5 24 points)
Write your answer on the answer blank provided.
11. Given that the ratio of boys to girls in a choir group is 17 : 23, what is the
relative frequency of the number of boys?
12. Solve the equation 3 ( 2x
4
5
x
3
5)
4.
13. A van uses 16.5 gallons of gas to travel 174.5 miles. How far can the van
travel on 22.4 gallons of gas? Round your answer to 3 significant digits.
14. In the diagram, BCXW is a parallelogram, m/WXB 5 34°,
and m/BCX 5 80°. Find the measures of /BXC and /ABW.
X
W
80°
C
B
A
15. A solid cone has a lateral surface area of 100U square centimeters
and a total surface area of 269U square centimeters. Find the base
radius of the cone.
152
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
© Marshall Cavendish International (Singapore) Private Limited.
34°
Name:
16.
Date:
The 100-meter backstroke swimming times, in seconds, for 8 swimmers are
as follows:
62.2, 55.3, 69, 72.1, 58.6, 60, 58.4, and 70
Find the mean absolute deviation of the times. Round your answer
to the nearest tenth.
17. In a piggy bank, 40% of the coins are dimes and three-eighths of the
remainder are nickels. A coin is randomly drawn from the bank. What is the
probability that the coin is not a nickel? Give your answer as a percent.
© Marshall Cavendish International (Singapore) Private Limited.
18. Simplify 2.8(3x 2 5y) 2 1.2(20.5 1 4x 2 2y).
19. Over a 4-month period, the total change in the average temperature
was 246°F. What was the average temperature change per month?
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
153
Name:
Date:
20. Using long division, write
125
as a repeating decimal in bar notation.
144
Solve. Show your work.
21. In the diagram, triangle XYZ is an enlarged scale drawing of triangle PQR.
Z
R
13.5 cm
7.95 cm
9 cm
9 cm
6 cm
154
4.5 cm
Q
X
Y
a)
Find the scale factor of the drawing.
b)
Find the perimeter of triangle XYZ.
c)
If the height of triangle XYZ is 7.95 centimeters, what is the height
of triangle PQR ?
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
© Marshall Cavendish International (Singapore) Private Limited.
P
Name:
Date:
22. In the diagram, triangle BDC lies inside of triangle ADC. Given that BQ is
parallel to CP, AB 5 BD 5 DC, m/BDC 5 54°, and m/ABQ 5 66°, what are
the measures of /DBC, /DBQ, and /ADC.
Q
P
D
54°
66°
A
B
C
© Marshall Cavendish International (Singapore) Private Limited.
23. The temperature recorded in Town A was 10°C at 12 P.M. The temperature was
recorded as –5°C at 8 P.M.
a)
Find the difference between these temperatures.
b)
Given that the temperatures in Town B and Town C at 12 P.M. are 12°C
and –5°C, find the average temperature of the three towns. Round your
answer to the nearest degree Celsius.
c)
If the temperatures in Town B and Town C decreased uniformly by 1°C per
hour, what is the average temperature of the two towns at 8 P.M.?
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
155
Name:
Date:
24. In the diagram; BC is parallel to FD , DC is parallel to GF and AD is parallel to
JK. Given that m/ABC 5 160°, m/CED 5 30°, and CE 5 DE, find the measures
of /CDE, /CBD, /BCE, /DFG, and /JFG.
C
160°
A
30°
B
E
F
J
D
K
G
156
a)
Write down an expression in terms of x for Christine’s age and her
father’s age.
b)
The sum of the ages of the four family members is 101. Write and solve
an equation for x.
c)
Find the mother’s age when Christine was born.
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
© Marshall Cavendish International (Singapore) Private Limited.
25. Leon is x years old. His sister Christine, is 5 years older than Leon. Their mother
is four times as old as Leon. Christine is one-third their father’s age.
Name:
Date:
A hemispherical candle holder is shown in the diagram. Use 3.14 as an
approximation for U.
26.
2 cm
5 cm
2.5 cm
a)
What is the total surface area of the candle holder?
b)
Find the volume of the candle holder. Round your answer to the nearest
tenth.
c)
A candle of diameter 4 centimeters and height 8 centimeters fits nicely
into the candle holder. Calculate the total surface area of the candle
that is exposed.
© Marshall Cavendish International (Singapore) Private Limited.
27. Rectangle ABCD has length AB 5 9 cm and width BC 5 4 cm.
a)
Construct rectangle ABCD. Construct the perpendicular bisectors
of the sides and label the midpoints as W, X, Y, and Z. Name the
type of quadrilateral WXYZ forms. Provide support for naming the
quadrilateral.
b)
Measure the perimeter and calculate the area of quadrilateral WXYZ.
Round your answer to the nearest tenth.
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
157
Name:
Date:
28. There are three different types of counters:
t 8IJUFPOPOFTJEFBOECMVFPOUIFPUIFSTJEF
t 8IJUFPOPOFTJEFBOESFEPOUIFPUIFSTJEF
t 8IJUFPOCPUITJEFT
The three counters are tossed together and the color each counter lands on
is recorded. (The order of appearance of the colors does not matter.)
a)
List all the possible outcomes.
b)
Construct a probability distribution table.
c)
Is the probability model uniform? State a reason.
Team A
a)
158
Team B
12
9
16
10
7
10
12
8
12
9
11
14
5
6
10
17
10
10
18
15
Calculate the mean number of airplanes folded by each team.
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
© Marshall Cavendish International (Singapore) Private Limited.
29. Two teams participated in a contest of folding paper airplanes. Below are the numbers
of paper airplanes folded by the participants of Teams A and B within a given time limit.
Name:
Date:
Calculate the mean absolute deviation for each team.
b)
c)
Which team has lesser variability in the number of airplanes folded?
d)
Which team performed better? Explain.
© Marshall Cavendish International (Singapore) Private Limited.
30. Below are the lengths, in centimeters, of 24 corncobs.
14.6
15.7
10.5
10.8
16.0
14.8
16.2
13.8
8.4
10.0
12.9
14.6
10.3
15.3
9.3
16.8
11.5
11.0
17.1
16.0
10.8
10.6
12.3
13.5
a)
Draw a stem-and-leaf plot of the lengths.
b)
Find Q1, Q2, and Q3 from the stem-and-leaf plot.
c)
Calculate the interquartile range.
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
159
Name:
Date:
31. The table shows the grade point averages (GPA) of 200 students in a certain high
school.
GPA
Number of Students
a)
1.0–1.9
2.0–2.9
3.0–3.5
3.6–4.0
24
73
85
18
Complete the following relative frequency table in percent.
GPA
1.0–1.9
2.0–2.9
3.0 –3.5
3.6–4.0
Relative Frequency
Represent the relative frequencies in a bar graph.
c)
If one of the 200 students is randomly selected, what is the probability that
the selected student has a GPA less than 3.0?
© Marshall Cavendish International (Singapore) Private Limited.
b)
160
End-of-Course Test A
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
Name:
Date:
Bonus Questions
Solve. Show your work.
32. There are 40 students using red and green beads to make a bracelet. 3 of
5
the students use red beads and 3 of the students use green beads. Some
4
students use both red and green beads to make the bracelet.
a)
What is the probability of selecting a student who chose red beads?
b)
What is the probability of selecting a student who chose both red and
green beads?
© Marshall Cavendish International (Singapore) Private Limited.
33. The number of hours, H, needed to assemble a part is inversely proportional
to the number of employees, w. A small factory has 12 employees working
6 hours a day. These employees can finish assembling the part in 5 days.
a)
Find the constant of proportionality for the inverse proportion.
b)
Write an equation that relates H and w.
c)
How many employees are needed to finish the same part in 9 days,
if they work 5 hours a day?
d)
If the factory pays each employee $15.50 per hour, find the total amount
of money the factory has to pay each employee for the 9 days of work.
Assessments Course 2
TRIAL MODE − a valid license will remove this message. See the keywords property of this PDF for more information.
161